Ground Zero fires could have heated the steel by up to 4 °F

Introduction

An abundance of forensic evidence proves the official 9/11 conspiracy theory is scientifically and physically impossible. If the official theory were true, the laws of thermodynamics and thermochemistry would need to be rewritten and people would survive months of burial in sand. Air flow through the Ground Zero pile is found to be more than three orders of magnitude short of that required to sustain smoldering combustion except for within voids, and sufficient to raise the temperature of the steel by 2.2 °C in five months. The maximum sustainable heat release rate is equivalent to one burning cigarette every 32 square feet. Steel that was heated to cherry-red would have cooled within hours.

The official conspiracy theory of nineteen Arabs with box cutters is easily refuted with a few short steps that evaluate the physics and the physical evidence. The death blow has already been dealt by combining 1) and 2) with 4) or 5) below, and several further points are provided for diehard reality deniers. A standalone set of evidence described in 8) is more than capable of rebutting the "Arabs did 9/11" thesis, and the same could be said of 6). Part 3) is necessarily lengthy, in order to dispel an aspect of the legend that is much misunderstood and has taken on a quasi-religious tone. Those who prefer to sidestep the (elementary) math can go on to part 4). Or go to the index to review a topic of interest.

What should not have been found at the WTC, if the official conspiracy theory were true

1) The official 9/11 narrative asserts that three steel-framed high-rises at the World Trade Center (WTC) collapsed following office fires in which no accelerants of any kind, e.g., thermite, were employed by the 9/11 conspirators.

2) The temperatures generated by office fires, such as those of 9/11/01 at the WTC buildings, are "most definitely not capable of melting steel", or iron, which has a melting point of 1,535 °C, or iron(III) oxide, which has a melting point of 1,565 °C. The various structural steels allowable for use in the WTC construction had a carbon content of up to 0.3% (e.g., the Japanese JIS G3101-73 grade SS50; see for example NIST NCSTAR 1-3A or its Interim Report Appendix E). The iron-carbon phase diagram shows that 0.3% carbon barely lowers the melting point to below that of iron. Given the steel's maximum sulfur content of 0.05%, its melting point was at least 1,426 °C or 2,600 °F.

Note that steel temperatures in office fires are always below the maximum (upper layer) gas temperatures. It takes time for heat to be transferred to the cooler, absorbing object, and the rate of heat transfer decreases as the temperature of the absorbing object approaches that of the heat source. NIST admitted that the "maximum upper layer air temperatures" would have been "about 1,000 degrees Celsius (1,800 degrees Fahrenheit) in the WTC towers", and also confirmed that "at any given location the combustibles needed about 20 minutes to be consumed". NIST said (NCSTAR1CollapseOfTowers, 2005 p.179) in reference to gas, not steel temperatures: "At any given location, the duration of temperatures near 1,000 °C was about 15 min to 20 min. The rest of the time, the calculated temperatures were near 500 °C or below." That would make the mean gas temperature about 600 °C for a given point in the fire zone. A method outlined here for estimating the mean gas temperature from the heat flux predicted an average temperature of 627 °C. And according to a study commissioned by Silverstein Properties, the temperatures ranged from 750 °F to 1300 °F (about 400 °C to 700 °C).

3) Similarly, oxygen-depleted underground fires that have been smothered with tens of thousands of tons of concrete dust, hosed with three million gallons of water and exposed to another million gallons of rain all within the first ten days, and sprayed with thousands of gallons of the fire retardant foaming agent Pyrocool FEF - of which 500 gallons sufficed to extinguish the Nassia oil tanker fire within a mere twelve-and-a-half minutes - should not generate temperatures at or above the melting point of iron or steel and mysteriously continue to burn at very high temperatures for more than three months, unless accelerants such as thermite have been employed.

A report on tritium levels at the WTC states: "It was determined that 3 million gallons of water were hosed on site in the fire-fighting efforts between 9/11 and 9/21 (the day of the tritium measurement, sequences 6,7 in Table 1) (Headley, 2002). In addition, there were 2 episodes of rain during the same 10 day period after the attack: on 9/14 and 9/20,21 (NOAA, 2001), totaling 0.9 million gallons of water in the Bathtub area. Considering the neighboring area, one can take 1 million gallons from the rain. Therefore, a total of 4 million gallons of water percolated through the debris in the first 10 days and collected at the bottom of the Bathtub."

In all, thirty million gallons collected at the bottom of the Bathtub over the first ten days and flowed through the north PATH train tunnel to Jersey City, whereupon it was having to be pumped out at a rate of thousands of gallons per minute over a 12-hour day. The north PATH tunnel was "completely filled" at the low midriver point. The source of the extra 26 million gallons was assumed by the tritium report authors to have been leaks from the Hudson River and broken mains, whereas the engineer who oversaw the construction of the Bathtub believed that "most" of the total came from the "vast amounts of water that were poured onto the debris to extinguish continuing fires". Thus, the figure of 4 million gallons percolating through the entire height of the pile over ten days may be too low, but can be taken as a conservative estimate. Throughout that time, there is no doubt that the bottom of the Bathtub was a giant lake.

Two more points of interest from the tritium report are (i) the authors' honest assessment of the underground fires, which they described as: "smoldering fires of much lower temperature than the explosive and high-temperature fires up in the Towers (with the exception of possibly the WTC 7 fire)", and (ii) their estimate of 0.1 for the air porosity in the debris pile: "Considering that the Bathtub was at least 50% destroyed and filled with the debris from the buildings (Post, 2001c), one could assume its air porosity of 0.1 (note: for instance, a porosity of close packed spheres is 0.26)." They go on to assume an air porosity of 0.3 for the B6 level with the PATH tunnel, "since the damage was less".

(A word of warning: ignore any claims that you may see about "nuclear" demolitions, "directed energy" beam weapons, "holographic" planes, "TV fakery", or "lizards". Intelligence agency assets have been promoting those red herrings for years, in a desperate attempt to mislead truth seekers and to portray critics of the government-approved conspiracy theory as illiterate imbeciles and raving lunatics who are taken in by absurd nonsense. Not surprisingly, the disinformation agents never support their wild flights of fancy with any form of quantitative analysis. The 2011 Toronto Hearings on 9/11 examined what they termed "the best available evidence", which was a polite way of saying that they would not countenance junk science and hare-brained speculation about "nukes" and "missiles" that were "faked" to look like planes.)

So, the WTC office fires were "most definitely not capable of melting steel", and anyone who wasn't trying to delude themselves or others would accept that the underground fires were, at most, merely "smoldering fires of much lower temperature" than the WTC office fires.

Proponents of the official 9/11 legend have tried to create a mystique about fires that "raged for a hundred days" in the WTC debris pile. They employed the same tactic regarding the office fires, with wild, excited babble about "intense heat" from "the burning jet fuel" - ignoring the fact that it all burnt up within a couple of minutes. The mass burning rate for free burning pool fires is on the order of 50 grams per square meter per second, so the burn time in seconds of a 4,000 gallon, 12,400 kg pool fire is 12,400 / (0.05 × Area in m²), or in this case is equal to 248,000 s.m² divided by the area in square meters. If spread over all of a single floor, then the area, deducting 10% to allow for vertical shafts and structures, would be 3,617 m² and the burn up time 68.6 seconds. If spread over four floors, each covering a quarter of the floor area , the time would be the same; if spread more thinly, it would have burnt up in even less time.

And there is no great mystery about underground fires in the ruins of collapsed steel-framed skyscrapers. If such fires generate extraordinarily high temperatures, e.g. "more than 2,800 °F" or 1,538 °C according to daily thermal measurements taken by helicopter, and persist at very high temperatures for months on end despite being smothered with concrete dust and hosed with millions of gallons of water, it is not because of some malign influence of the evil spirits of "Muslim" terrorists. Any method of staging deceptive controlled demolitions that are designed to resemble fire-induced collapses will leave some telltale signs. The best, stealthiest method would employ some variant(s) of thermite and leave evidence of very high temperatures, which the perpetrators would hope to blame on the "intense heat" from "the burning jet fuel". In the thermite reaction the aluminum grabs pure oxygen from the iron oxide. Thus, thermite not only has its own oxygen source and cannot be extinguished by water, it can generate temperatures well in excess of those associated with hydrocarbons burning in air of up to 21% oxygen.

Controlled demolitions that relied upon conventional "bombs" or high explosives would be all too obvious, and it would not take long before the perpetrators were brought to justice. Deceptive demolitions based on thermitic materials offered the conspirators the best prospect of getting away with the crime for the remainder of their lives, notwithstanding the fact that in an age when information could be freely exchanged via the internet, it would take no more than ten years before structural engineers, architects, physicists, mechanical engineers, metallurgical engineering graduates, PhD scientists, fire protection engineers, fire-fighters, electrical design engineers, explosives technicians, psychologists, etc., were explaining why the official conspiracy theory was believable only to the ignorant, the indifferent and the ill-informed. And various experts would tear apart the wild claims and speculation of the government account at an international hearing in which the panelists were a top judge and various professors.

Proponents of the official conspiracy theory claim that thermite would have all burned up in minutes. However, the official theory not only fails to account for the observed very high temperatures, it also flounders in its attempts to explain the observed long-lasting fires. As will be shown, even smoldering fuel would burn up within an hour to a day, and so the official theory requires an extraordinary series of local burning of pulverized office materials under soaking, smothered conditions followed by transmission of heat that improbably ignites soaked, smothered office materials elsewhere, with the cycle necessarily repeating hundreds or even thousands of times over the following months, and with dozens of these series running in parallel. Under smothered, soaking conditions, no more than a tiny proportion of the office combustibles could continue to smolder. In contrast, accelerants used for the controlled demolitions could continue to burn in water and without an independent supply of oxygen, going on to generate extraordinarily high temperatures, with this heat subsequently igniting accelerants elsewhere by various means.

Paint chips on rusty steel from the WTC were found to be contaminated with an accelerant that included elemental aluminum as the fuel. Iron spheres were generated when the chips were heated to 700 °C, indicating flame temperatures above the melting point of iron. Such a material would be ignited at irregular intervals whenever the clean-up brought it into contact with cooling zones that had been superheated from thermite that had already burned elsewhere, or when its molten iron product flowed down into the pile in the vicinity of unreacted nanocomposites, or after sufficient heat had been conducted through the concrete or steel. Some active accelerant would be ignited soon after the collapses by smoldering office combustibles. The vast majority of the time, the thermite is not burning; it has been consumed in one part of the pile and there is a delay before heat has been conducted over the required distance through the concrete or steel, or the molten iron flows down through the pile to ignite active thermitic material elsewhere, or mechanical diggers bring unreacted thermite into contact with debris that is still at a few hundred degrees or more. Then the next zone is ignited to form another superheated area, and the cycle repeats.

An Al-Fe₂O₃-based energetic nanocomposite using nanowires embedded in a 50 nm aluminum film was found to ignite at 410 °C and to produce a flame temperature of ~4,000 °C, which is even higher than the theoretical adiabatic flame temperature of 3,250 °C for a stoichiometric Al-Fe2O3 mixture. The flame temperature was measured by recording the blackbody spectrum from the light flash. It is believed that the reaction is so fast in this material that the heat is unequally distributed amongst the products.

As will be demonstrated, office combustibles relying upon the available oxidizer flux within the pile would have been capable of raising the temperature of the steel by a mere 2.2 °C. And that's assuming all of the heat remained trapped in the pile and continued to build up for a full five months, and all of this heat was transferred to the steel rather than the concrete, aluminum or glass, etc. If we include the requirement to heat the concrete, the potential temperature increase is only 0.7 °C - barely more than 1 °F. Energetic nanocomposites provide the only plausible explanation for the phenomenon of mysteriously persistent fires that generated extraordinarily high temperatures along with a molten iron product that was frequently seen "dripping", "flowing", and "running", e.g., "down the channel rails". To go straight to one of the main conclusions for this section, click here; to see how it was arrived at, read on.

According to NIST, a typical WTC floor had 4 psf of combustible materials. If this is applied to 208 by 208 feet, which is 43,264 square feet, each floor contained 173,056 pounds or 86.5 tons of combustibles such as paper, carpets and workstations. (It also had ~56 tons of fireproofing.) Thus, 110 floors above grade and six below contained 10,037 tons of combustibles - say, 10,040 after allowing for those from the plane, e.g., seats. The oft-quoted 500,000 tons per tower is a high estimate that assumes the live load is almost 100% of the design maximum, and so the combustibles constituted up to 3% of the WTC debris pile by mass. That pile mostly consisted of concrete dust and steel members, along with aluminum window panels, glass and sprayed fire-resistive material - and plenty of water percolating through it. Moreover, at the time of collapse most of the floors were not affected by fire, leaving the vast majority of combustibles located on floors much lower (or higher in the case of WTC7) than the burning floors as the buildings collapsed. This potential fuel was therefore cold and isolated from hot spots in the pile. Other combustibles had already been consumed, and the few remaining pockets of burning paper, carpets or workstations - around 0.1 to 0.2% of the total mass of the pile - would have been isolated from other fuel sources. The pile was subjected to "a nearly constant jet of water" that effectively created a "giant lake".

FEMA's report on WTC1 and 2 quoted Culver's findings that typical office-type occupancies had fuel loads, expressed in terms of the equivalent weight of wood, ranging from 4 to 12 psf with the mean slightly less than 8 psf. NIST's 4 psf is lower than the Culver figure because the calorific value of wood, at up to 16 - 17 MJ/kg, is less than that of other combustibles such as plastics. It is also necessary to allow for the fact that the effective heat of combustion in ventilation-limited fuel-rich compartment fires is around 35% lower than could be obtained under an optimum (stoichiometric) fuel-air ratio that allowed for complete combustion. Kawagoe and others such as Magnusson and Thelandersson used a value of 10.78 MJ/kg as the calorific value of wood in fully developed ventilation-limited fires.

Thus, a fire load of 8 psf or 3.63 kg per square foot of wood in a compartment fire yields some 3.63 × 10.78 = 39.1 MJ per square foot or 421 MJ/m² or 1.69 TJ over the 208 x 208 feet of a WTC tower floor. If this energy was all released over the typical 20 minutes (as quoted by NIST) for local combustibles to be consumed in a compartment fire before the flames move along to a fresh fuel source, the power output would be an impressive 1.69×10¹² / (20 × 60) = 1.41 GW per floor, or 32.6 kW/ft² or 351 kW/m². (About 40% of that is vented out and drives the smoke plume - FEMA quoted "one third to one half".)

However, if the energy is released over 100 days instead of 20 minutes, the mean power output decreases by a factor of 7,200 to 48.7 watts per square meter, which is less than half of the 120 W/m² required to qualify for the World Meteorological Organization's definition of sunshine, and more than an order of magnitude below the 1 kW/m² received at a point in the tropics when the sun is directly overhead on a clear day. Even if we allow for each floor being compressed into about eight inches, the equivalent of hazy sunlight shining on eight inches of mostly concrete dust is not going to ignite wood, plastics, etc at a depth of a few inches.

And those very high temperatures in the pile were in fact sustained for five months, according to Bronx fire-fighter Joe "Toolie" O'Toole. He reported seeing a crane lift a deeply buried steel beam that was "dripping from the molten steel" as late as February, 2002.

An oxygen-starved, smoldering underground fire would be even less efficient than a fully-developed ventilation-limited compartment fire. The assumption of 65% efficiency and 10.78 MJ/kg of wood in a compartment fire is consistent with an averaging of a range of equivalence ratios from 1 (stoichiometric) to 2 (fuel-rich) at various points within the compartment - from relatively complete combustion near the vents to incomplete elsewhere. (Integrating the range gives a mean equivalence ratio of 1.386 - the natural log of 4.) The smoldering underground fire would be expected to involve more incomplete combustion, with a greater proportion of CO product in place of CO₂. The ratio of CO₂ to CO in smoldering is typically around unity, compared to about ten times as much CO₂ in a fully developed compartment fire. However, for the Ground Zero fires, the most important figure is the heat released per unit of oxygen consumed.

Flaming combustion of typical hydrocarbons is associated with a heat release of around 13.1 kJ/g of oxygen consumed, whereas the corresponding value for smoldering combustion is about 5 kJ/g of oxygen (5,000 kJ/kg). Bar-Ilan et al (2004) obtained 5,880 kJ/kg-O₂ for opposed (reverse) smolder and 4,550 kJ/kg-O₂ for forward smolder, compared to the 3,900 kJ/kg-O₂ of Torero et al (1993) and 4,550 kJ/kg-O₂ of Walther et al (1998).

Smoldering combustion is typically self-sustained at heat fluxes around 6.5 kW/m² or more. Bar-Ilan et al (2004) found that, given their sample size and test conditions of forced air mass fluxes, "there exists a minimum critical oxidizer mass flux to achieve a self-sustaining smolder propagation". They placed the value of this minimum mass flux at around 0.6 grams of oxygen per square meter per second under normal gravity. (The minimum flux was only half as much, 0.3 g/m².s-O₂ under microgravity conditions, apparently because "the presence of buoyant heat losses in normal gravity hinders the smolder reaction".) At 5 kJ/g of oxygen consumed, an oxidizer mass flux of 0.6 g/m².s-O₂ would correspond to a power density of 3 kW/m².

Dr. T. J. Ohlemiller (of NIST, ironically) says that a reverse smolder front of a few kW/m² from a source 0.1 to 0.15 m. in diameter would typically generate gas temperatures of 670 to 970 K (400 to 700 °C), and would be so "weak" that the buoyant plume might not even reach the ceiling of a room.

According to Darcy's law, the total discharge, Q (units of volume per time such as m³/s), of fluid through a medium is equal to the product of the permeability of the medium, k (m²), the cross-sectional area to flow, A (e.g. m²), and the pressure drop, P1 - P2 (Pa), all divided by the viscosity, µ (Pa.s) of the fluid and the length, L (m) that the pressure drop is taking place over.

Q = -k × A × (P1 - P2) / (µ × L)

The permeability k of beds of close packed spheres can be predicted from the porosity and the sphere diameter. Dr. Frank Greening estimates the distribution of particle diameters of the pulverized WTC concrete as 30% within the range 10 cm to 1 cm, 20% within 1 cm to 1 mm, 15% within 1 mm to 100 µm, 10% within 100 µm to 10 µm, and 25% at less than 10 µm. Taking the mean of the logs of each size range in microns (and ignoring the nm range), we have 30 × 4.5 + 20 × 3.5 + 15 × 2.5 + 10 × 1.5 + 25 × 0.5 totalling 270, which is divided by 100 to give the mean 2.7, which places the typical particle diameter at around 500 µm.

This is consistent with sand, of which 0.25 to 0.5 mm grains is classed as "medium" sand, and 0.5 to 1 mm is "coarse" sand. The WTC concrete dust's range of sizes includes all of the silt range (0.0625 mm down to 0.004 mm diameter) and all of gravel (2 mm up to 64 mm). Given the large range of sizes, 0.1 is a fair estimate for the WTC porosity. The smaller particles fill the voids between larger particles, whereas with spheres of equal size the maximum packing density is about 0.74.

Both the Carman-Kozeny model and Rumpf and Gupte's equation predict the permeability k (m²) by squaring the sphere diameter d (m) and then multiplying by some terms that incorporate the porosity (dimensionless, denoted by epsilon ε or phi φ). Carman-Kozeny have d² multiplied by ε³ / [180×(1-ε)²] which in the case of ε=0.1 is d² times 6.859×10^-6. In Rumpf and Gupte's equation d² is multiplied by (ε^5.5) / 5.6 which is d² times 5.647×10^-7. It is said that the Rumpf-Gupte equation "replicates better experimental data". Nevertheless, let us use the Carman-Kozeny model, which predicts a higher permeability and thus is more favorable to the official 9/11 conspiracy theory. So for a mean particle diameter of 0.5 mm, as derived from Greening's distribution of particle sizes, the permeability of the WTC concrete dust in the pile is 0.0005² × 6.859×10^-6 = 1.71×10^-12 m² or 1.71×10^-8 cm². This is well within the range of sand, rather than gravel or silt.

Source: Associated Press

And it is consistent with photographic evidence showing the pile to have the consistency of sand, with a few larger pieces mixed in.

According to Table 1 in the Lioy et al report on the WTC Dust/Smoke Aerosol, the aerodynamically separated samples ranged from 52.21% to 63.6% by mass of particles greater than 53 µm diameter, and the sieved samples had 16% to 23% by mass of particles greater than 300 µm diameter. This suggests that the typical dust particle was smaller than 500 µm diameter, which would make the permeability lower than 1.71×10^-12 m². It's still consistent with poorly-sorted sand of low porosity.

Given the permeability, we can now calculate the Darcy velocity and discharge rate for various fluids such as water and air.

Q = -k × A × (P1 - P2) / (µ × L)

The total discharge rate through a given cross-sectional area is directly proportional to the permeability, the area and the pressure drop along the length of the porous medium under consideration, and inversely proportional to the dynamic viscosity of the fluid and the length of the medium. Dividing the pressure drop (P1 - P2) by the length L gives the pressure gradient or the change in pressure per unit length ΔP, and dividing both sides by the area gives the discharge or flux per unit area. So Darcy's law can also be written as:

q = -k × ΔP / µ

...where q is the discharge per unit area (m/s), k is the permeability of the medium (m²), ΔP is the pressure gradient (Pa/m), and µ is the dynamic viscosity of the fluid (Pa.s). This discharge per unit area is the Darcy velocity, and is equal to the total discharge rate in cubic meters per second for a 1 m² cross-sectional area. For a porosity of 0.1, the real velocity of the fluid through the pores is ten times greater than the Darcy velocity.

In the case of a gravity-driven downward flow, the pressure gradient derives from the fluid density (and from the gravitational acceleration constant). For a hydraulic head of water, each foot of height gives about 0.43 psi of pressure, irrespective of the area of the pipe or dam. A pipe of 1-meter cross-section filled to a height of 1 meter contains 1 m³ of water, which has a mass of 1,000 kg. The gravitational force acting on the 1,000 kg that would cause it to accelerate in freefall at 9.802 m/s² (in New York City) is 9,802 N, and so the pressure acting at the bottom is 9,802 N/m² = 9,802 Pa. (Dividing the 1,000 kgf by 3.2808 to convert to a height of 1 foot, dividing by 39.37² to convert to a 1 in² area, and multiplying by 2.2046 to convert to lbf gives the 0.43 psi for a 1 ft head of water.) Thus, the pressure gradient ΔP is 9,802 Pa/m.

The dynamic viscosity of water at 10 °C is 0.001308 Pa.s. Thus, we now have the necessary variables to calculate the Darcy velocity q, which is equal to the permeability 1.71×10^-12 m² times the pressure gradient 9,802 Pa/m divided by the dynamic viscosity 0.001308 Pa.s, giving the Darcy velocity as 1.281×10^-5 m/s. The total discharge rate for a 1 m² area is 1.281×10^-5 m³/s, and the actual flow velocity in the 10% spaces between the spheres is 1.281×10^-4 m/s. This is not far off the approximation shown on this page of 2 hours for water to travel 1 meter down through sand, which is a rate of 1.389×10^-4 m/s.

The known flow of four million gallons through the Bathtub in ten days can be used to set a lower limit for the permeability at Ground Zero. The Bathtub area was 510 ft by 980 ft, which is 46,434 m². Four million gallons is 15,100 m³, and that volume over ten days is equal to 0.0175 m³/s. Rearranging:

Q = -k × A × ΔP / µ

...gives

-k = Q × µ  / (A × ΔP)

...and so the permeability k is a minimum of 0.0175 × 0.001308 / (46,434 × 9,802) = 5.03×10^-14 m² (or 34 times lower than the 1.71×10^-12 m² obtained above). This would correspond to a typical particle diameter of 0.5 mm / √(34) = 86 µm.

At a density (and thereby pressure gradient) some three orders of magnitude lower than water and a dynamic viscosity around two orders of magnitude greater than water, the Darcy flow and velocity for air is about one-tenth that of water. Moreover, any air that might flow down into the WTC "sand" not only meets resistance from the solid spheres, but from water and foam being hosed onto the pile. The water flows along the surface of the solids, thus coating the combustibles as it is held by surface tension, whereas the air flows between the outer surfaces of the water, isolated from the combustibles.

For air, the pressure difference per meter of height is the gravitational acceleration constant 9.802 m/s² times the density of air, which is 1.247 kg/m³ at around 10 °C for 1 atm. So each meter height of air has a mass of 1.247 kg for every square meter, and gravity acting on its mass gives it a weight of 1.247 kg/m². The 1.247 kgf per m² translates to 2.749 lbf or 12.22 N, and a pressure of 12.22 N/m² is 12.22 Pa. Thus, the pressure gradient ΔP for air is 12.22 Pa/m. (At 20 °C air is less dense, so the pressure gradient is only 11.76 Pa/m.) And the dynamic viscosity for air at 10 °C is 1.787×10^-5 Pa.s.

From:

q = -k × ΔP / µ

...we have a value of 1.71×10^-12 m² × 12.22 Pa/m / 1.787×10^-5 Pa.s to give a Darcy velocity of 1.169×10^-6 m/s, a discharge rate of 1.169×10^-6 m³ for each square meter cross-section, and an actual velocity in the pores of 1.169×10^-5 m/s. To obtain the air mass flux we multiply the discharge rate by the density of air of 1.247 kg/m³, which gives 1.458×10^-6 kg/m².s-air or 0.001458 g/m².s-air. Given that the oxygen proportion is 23.2% by mass, the mass flux of oxygen available is 0.000338 g/m².s-O₂, which at the oxygen heat release of 5 kJ/g-O₂ for smoldering combustion could support a power density of 1.69 W/m².

This is more than three orders of magnitude short of the minimum 0.6 g/m².s-O₂ for self-sustained smoldering, which is associated with a power density of 3 kW/m².

The pressure gradients in air tend to be greater in the horizontal plane, resulting in the winds and breezes that are normal meteorological phenomena. By rearranging:

q = -k × (P1 - P2) / (µ × L)

...to get:

P1 - P2 = q × µ × L / -k

...we can find what pressure difference would be required to force air through the pile formation at a discharge rate that might sustain smoldering combustion, if for example there was a higher atmospheric pressure over Jersey City that might have forced air through the south PATH tunnel - which apparently wasn't completely flooded - and up through the pile and out into Manhattan. (We don't enter in the area, as we are interested in the Darcy flow per m², but we put in the length of the formation over which the pressure drop occurs. This will take account of the required pressure gradient, which needs to be about 1,775 times greater than 12.22 Pa/m, i.e., 21,690 Pa/m, which is ominously high!)

The required oxidizer mass flux is 0.6 g/m².s-O₂, which requires 0.6 / 0.232 = 2.59 g/m².s-air, which requires an air discharge rate of 0.00259 kg / 1.247 kg/m³ per second per square meter = 0.00207 cubic meters of air per second per square meter, or a Darcy velocity of 2.07×10^-3 m/s. The length (height) of the formation is taken as 21.3 m (70 ft).

So the required pressure difference P1 - P2 is 0.00207 m/s × 1.787×10^-5 Pa.s × 21.3 m / 1.71×10^-12 m² = 460,764 Pa. This is 4,608 mbar, 4.547 atm, or 66.83 psi. Such a pressure difference would lead to some strong winds above grade, to say the least!

In fact, the WTC towers would probably have been the only buildings in Manhattan and Jersey City that could have survived such winds, since they were designed to withstand hurricanes, earthquakes, and a 600 mph impact with a jetliner that dumped all of its fuel into the building creating a "horrendous fire" that "killed" a "lot of people" but left "the building structure" in place. The exterior columns had "tremendous reserve strength", in that "live loads on these columns" could "be increased more than 2,000% before failure" occurred. Even the infamous Popular Mechanics publicized the fact that the WTC would "withstand winds up to 150 mph with no ill effects", although their moments of candor were in 1986 and 1993, long before the need for a massive cover-up.

Darcy's law may be applied to fluids including water or air, provided the Reynolds number is not too high, which would indicate turbulent rather than laminar flow. In the case of flow of fluid through a bed of approximately spherical particles of diameter d in contact, taking the voidage as ε and the (superficial) Darcy velocity as q, then a Reynolds number can be defined as:

Re = ρ × q × d / (µ × (1 - ε))

For the above case of water with a density ρ of 1,000 kg/m³, a predicted Darcy velocity q of 1.281×10^-5 m/s, a mean particle size d of 0.0005 m, a dynamic viscosity µ of 0.001308 Pa.s, and a voidage or porosity ε of 0.1, plugging in the variables gives Re = 0.00544. For air of density 1.247 kg/m³, predicted Darcy velocity of 1.169×10^-6 m/s and dynamic viscosity of 1.787×10^-5 Pa.s, then Re = 0.0000453. Laminar flow applies up to Re = 10, and fully turbulent flow applies for Re = 2,000 and up. Thus, laminar flow holds and Darcy's law is applicable in both cases.

The predicted oxidizer mass flux through the WTC pile, at 0.000338 g/m².s-O₂, is short of 0.6 g/m².s-O₂ by a factor of 1,775. The 0.6 g/m².s-O₂ is required to obtain a bare minimum 3 kW/m² smoldering power density, which would still be too low to sustain smoldering for a range of potential fuel. And the Rumpf and Gupte model's prediction falls short by an additional factor of 12!

Since the permeability is proportional to the particle diameter squared, a 42-fold increase in d would be required to raise the permeability to around the required (minimum) value. This would place the mean particle diameter at 21 mm or 0.83 inches and squarely into the category of pebbles, even coarse gravel, which it clearly was not. The dust was reported to be "like a powder similar to baking flour". Since there was a wide range of grain sizes with finer particles inevitably filling the gaps between coarse particles, it was the very antithesis of well-sorted. Thus, it had low porosity, and was likely to have a lower permeability than well sorted sand.

Let's suppose for a moment that the fuel in the pile was somehow capable of sufficient air intake and exhaust output to continue burning for months.

As previously shown, the fire load of 8 psf of wood equivalent fuel per floor of a WTC tower translates to 39.1 MJ/ft², which is 421 MJ within a particular square meter zone of a compressed tower floor. A typical heat release rate for smoldering is 6.5 kW/m², so if we take that as a typical heat release rate for pieces of smoldering fuel within an oxygen-poor debris pile fire, then the 421 MJ/m² WTC fuel load would typically be consumed in 421,000 / 6.5 = 64,769 seconds which is ~18 hours, for any given location. The 20 minutes typical burn up time for local combustibles in an office fire has increased by a factor of 54, which is the ratio of the 351 kW/m² flaming combustion of the office fire to the 6.5 kW/m² smoldering fire. (An analysis below based on sizes of fuel shows that burn times could range from more than a day down to seconds, since the combustible materials were pulverized along with the concrete. Thus, although the largest pieces could smolder for over 18 hours, the 18 hours is associated with relatively large pieces of fuel in a particular zone.)

Since the heat release rate is on the order of 6.5 kW/m² for all of these hypothetical smoldering pockets of fuel in the months following 9/11, the time for fuel to be consumed at any given point remains the same irrespective of our arbitrary area of the block under consideration. Rather like the office fires that have any given point burning out after 20 minutes, the time typically taken in the smoldering case is ~18 hours, if all fuel in the block is assumed to have started burning at the same time. And in fact, the first pockets of smoldering fuel would have already been part consumed in the office fires before collapse, so this fuel would have burned out in even less time.

Thus, the official theory requires a bizarre scenario of localised pockets of soaking, smoldering office combustibles that burn at around 6.5 kW/m² whilst enclosed within concrete dust, and then these smothered, drenched, Pyrocool foam-treated pieces of burning paper, plastic or carpet somehow manage to transfer sufficient heat to ignite adjacent pockets of buried plastic or carpet in a wet, oxygen-depleted environment, with this most improbable ignition process occurring not just once, but hundreds or thousands of times over the course of five months throughout the debris pile, continuing to generate temperatures well in excess of the typical 600 °C associated with smoldering combustion. (And these bits of smothered wet paper, etc., have enough energy left over to partly evaporate steel and heat it to cherry-red, and leave such an abundance of "flowing", "dripping" molten steel or iron and possible other metals that the melt forms "little rivers" and looks "like a foundry"!!!)

A typical series of one smoldering pocket igniting an adjacent pocket of combustibles would need to involve ~200 instances of heat transfer and ignitions over five months. Given the persistence of dozens of hot spots at various points in the pile for months on end, there would have to be dozens of parallel sequences that included a similar series of ignitions. If fresh combustibles were enclosed within the same void as already smoldering fuel, heat could be transferred via the hot gases within the void. However, these would be expected to ignite and smolder at roughly the same time as the original burning fuel. Thus, subsequent series of heat transfer and ignition would be as a result of conduction through the concrete dust or the steel, which leads to some interesting calculations on how far sufficient heat could be conducted to ignite adjacent pockets of fuel before fuel in one zone burned out and the source began cooling. The answer turns out to be: not very far!

Of course, there was not sufficient air intake and exhaust to oxidize the fires; at least, nothing beyond a paltry 1.69 W/m², equivalent to a reasonably sized mono AM radio or a third of a burning cigarette per square meter. The fact that fires are extinguished by smothering in non-combustible material such as sand or pulverized concrete (whether or not they're soaked with water and treated with foam), and the fact that this is confirmed by calculation of the Darcy flux rate for air flowing through the medium, is corroborated by the fact that humans do not survive weeks or months of burial in sand.

For smaller 0.01 m² blocks of 0.1 x 0.1 meters within the collapsed towers' footprints, the heat release rate is typically 0.01 × 6.5 kW = 65 W per block. At the same power density, but with one-hundredth the fuel of a 1 m² block, the fuel in the smaller block is consumed in the same time, at 4.21 MJ per 0.01 m² divided by 65 W = 64,769 seconds which is ~18 hours. That's if it has oxygen!

Each 0.1 x 0.1 x 0.2 m pocket that is smoldering at 65 W has a volume of 2 liters, and given the porosity of 0.1 in the debris pile, had up to 0.2 liters of air (after allowing for water and Pyrocool foam). At 20 °C (sea level) the density of air is ~1.2 grams per liter, so 0.2 liters of air weighs 0.24 grams. Since air is 23.2% oxygen by mass, the 0.24 g of air contains 0.056 g of oxygen. Assuming a heat release in smoldering of 5 kJ/g of oxygen consumed, and with 65 J released each second, then the oxygen required each second is 65 / 5,000 g = 0.013 grams. Thus, the 0.056 g of oxygen would be consumed in ~4.3 seconds. Thereafter the Darcy discharge rate of 1.169×10^-6 m³ for each square meter cross-section provides 0.000338 g/s of oxygen per m², which is 3.38×10^-6 g/s of oxygen for the 0.01 m² block. (And even that's supposing that the fresh air could rapidly diffuse horizontally through the dust, which it couldn't. Even at the vertical Darcy velocity of 1.169×10^-6 m/s, it takes 5.94 hours to travel 0.025 m.) The fire is extinguished.

In comparison, the power output of a human being is on the order of a 100 watt light bulb. The human averages about 15 breaths a minute, and exhaled air has about 4 to 5% less oxygen than inhaled air. Thus, if each breath averages 500 mL of air, the volume of oxygen consumed is around 0.5 × 15 × 0.045 = 0.337 liters per minute. The density of oxygen gas at 20 °C and 1 atm is 1.331 grams per liter, so the 0.337 liters / minute is ~0.45 grams / minute or 0.0075 grams / second of O₂. The human uses oxygen more efficiently than the smoldering fire, since inhaled air is rich in oxygen and the combustion products are CO₂ and H₂O rather than CO and H₂. So if the human achieves around the 13.1 kJ/g of oxygen associated with complete combustion, the energy released each second is ~98 J; a power output of 98 W.

The average human has a mass of about 70 kg and a density around that of water, placing the volume of the average human at 70 liters. And the average body surface area of an adult human is 1.73 m². Fortunately, the "light bulb" human is of course oxidising much more slowly than the typical smoldering rate of 6.5 kW/m², especially when considering the additional area of internal organs.

Being buried alive has been used as a form of execution, murder, torture, and genocide of those deemed to be "sub-human". Without a fresh air supply, the individual would be breathing air with insufficient oxygen and too much CO₂ within 1 to 2 hours (if buried in a coffin thereby providing an initial volume of air), and death would follow within minutes. Harry Houdini was almost killed in one stunt involving burial under six feet of earth without a casket, and in another attempt managed to remain in a sealed casket submerged in a swimming pool for an hour and a half. A consumption of 0.337 liters of O₂ per minute would total around 40 liters in two hours. The person would be dead before the oxygen had run out, due to high CO₂ levels. Similarly, a smothered fire will extinguish itself before the oxygen gets down to zero. A match would not stay alight below about 15% oxygen. The products of combustion - CO₂ and H₂O - are used in fire extinguishers, and the bucket of sand is another useful extinguishing method.

For each person who was buried alive, there would need to be sufficient air permeability to allow at least 20 liters of oxygen per hour to reach them, whereas each 0.01 m² smoldering pocket requires 0.013 grams which is 0.0098 liters per second or 35.2 liters of oxygen per hour.

Let's pretend again for the moment, for the sake of argument, that the office combustibles did receive enough oxygen.

If we consider an "average" cubic meter within the debris pile, it turns out to have around 97.65 kg of actual combustibles, 965 kg of steel and 1.18 tonnes of concrete. Each tower's footprint was 208 by 208 feet or 63.4 by 63.4 meters, which is 4,019 m². Each compressed floor is assumed to be 0.2 meters, giving a total depth for 116 floors of 23.2 meters; thus the total volume is 93,240 m³. (In the previous calculation on the pressure difference required to force air from Jersey City and the PATH tunnel up through the pile, the height of "the formation" is taken as 21.3 m (70 ft). The 0.2 m per floor here is an approximation, but two or three meters can be allowed for the depth of the flooding at the bottom of the Bathtub at the B6 level, as tens of millions of gallons leaked from the river and from water mains. The tritium report assumes about ten feet of flooding, since it says that "the hose and rain water flowed" through "6/7 of the void volume of the Bathtub".)

Each tower had about 110,000 tonnes of concrete and 90,000 tonnes of steel, leaving 110,000 / 93,240 tonnes = 1.18 tonnes of concrete and 90,000 / 93,240 tonnes = 965 kg of steel per cubic meter in the pile. For the actual combustibles, we take the NIST figure of 4 psf, and allow double the 10.78 MJ/kg associated with FEMA's or Culver's 8 psf of fuel expressed as the equivalent weight of wood. So 4 psf is 19.53 kg/m² (for one floor). The 1 meter depth is five compressed floors, making the total combustibles 97.65 kg within the meter cube, and at 21.56 MJ/kg, the total potential heat release from the fuel is an impressive 2.105 GJ.

Taking specific heats of 800 J/kg for the concrete and 550 J/kg for the steel (which allows for higher values at higher temperatures), the energy required for each degree Celsius increase in the steel plus the concrete in the 1-meter cube is:

800 × 1,180 + 550 × 965 = 1.475 MJ.

(For simplicity, and to be fair to the government's conspiracy theory, we ignore the aluminum, glass, fireproofing, air conditioning and other loading. This places the combustibles at over 4% of the total mass, when in fact they were 3% or lower.) Thus, the potential temperature increase for the concrete and steel within the one-meter cube is 2,105 / 1.475 = 1,427 °C.

Of course, such temperatures would not be reached, partly because office fires do not get up to such a temperature, and partly because smoldering combustion temperatures are only around 600 °C, even when we ignore the fact that the fuel in the debris pile was too starved of oxygen to support even smoldering. A good supply of pure oxygen could generate steel-melting temperatures - but then the situation is getting close to a thermite scenario. Fuel is necessary, but not sufficient, for combustion. This is fortunate for life on earth, since there is a tremendous fuel load on the earth's surface, most of which isn't burning at any given time. (Although such a benign state of affairs may not endure, if the murderous, unscrupulous psychopaths behind 9/11 are allowed to carry on pulling the strings of puppet presidents and puppet prime ministers for much longer.)

As the fire triangle tells us, "Without sufficient heat, a fire cannot begin, and it cannot continue", and "Without sufficient oxygen, a fire cannot begin, and it cannot continue".

If 1% (a high estimate) of the debris pile was initially at 1,000 °C and the remainder at 10 °C, then the mean temperature within the pile was initially at (1,000+99×10)/100 = 19.9 °C.

Given the typical time to burn up when smoldering of 18 hours, the proportion of the fuel that was smoldering at any particular time could be no more than ~0.5% in order to sustain fires that persisted for five months. If all of the fuel in a 1 m³ volume of the pile was burning at the same time (which it wasn't because of lack of oxidizer flux), then no more than a few hundred out of these 93,240 one cubic meter volumes could be smoldering. Or alternatively, 1/200 of the fuel could have been burning at any particular time within each 1 m³ volume, moving on to adjacent fuel hours later. (As will be seen, even this could not be sustained.) Thus, if the hot spots are at 600 °C and the cold spots are at 10 °C, then the mean temperature across the debris pile cannot be any higher than (600+199×10)/200 which is about 13 °C.

Or that would be 3 °C, when the cold spots were at freezing point. That is inconsistent with the experiences of those who were actually at the site and found that "on the cold days, even in January, there was a noticeable difference between the temperature in the middle of the site than there was when you walked two blocks over on Broadway", and "You could actually feel the heat". Three degrees, a "noticeable difference" that you could "actually feel"?

(The government loyalist may cry, "But I don't care about the average temperature - some of those burning zones could still have been very hot, even if they were only 0.5% or whatever of the pile. Think of all that fuel, and how the heat was trapped in." Never mind, the temperatures go down even lower when we take account of the lack of oxygen. And this time, they are all low!)

If we pretend the impossible occurred, then occasionally there might be a group of adjacent 1-meter cubes that were smoldering. However, after eighteen hours, these would have burned up and the whole zone would cool towards the mean pile temperature. The great majority of the time, a few little bits of burning paper or carpet, etc., would smolder away for hours in some isolated 1-meter block, with the adjacent blocks barely above ambient temperature. When all fuel in the smoldering block had burned up, its heat would be conducted away in both directions along each of the x, y and z axes. A temperature increase of less than one-seventh of 1,427 degrees (204 °C) in adjacent blocks would exceed the auto-ignition temperature of hardly any potential fuel, making even a much smaller than 200 series of conductions and ignitions highly improbable.

Thus, even when we pretend the impossible was possible, the government account remains highly improbable. And when we take into account the lack of oxygen and the abundance of water, in reality these bits of burning paper or carpet would not be smoldering, making such a five-month sequence physically impossible.

So how much air or oxygen would be required to support temperatures of around 600 °C in the steel and the concrete? For a 600 degree temperature increase in the steel and the concrete in the average 1-meter cube, the energy required is 600 times 1.475 MJ, about 885 MJ. Each cubic meter of air has a mass of 1.2 kg and an oxygen content of 0.232 × 1.2 = 278.4 grams. Each gram of O₂ releases 5 kJ in smoldering combustion, so each cubic meter of air can release 278.4 × 5 kJ = 1.392 MJ. Thus, 1 m³ of air would be sufficient to raise the temperature of the concrete and steel by 1.392 / 1.475 = 0.944 °C, and the volume of air required to support a 600 degree increase in the steel and the concrete is 600 × 1.475 / 1.392 = 885 / 1.392 = 636 m³ or 636,000 liters of air.

Since the volume of air required is 636 times the volume of the pile that is to be heated by 600 degrees Celsius, the volume of air required to raise one tower's entire pile by 600 degrees is 93,240 × 636 = about 59,300,000 m³, which is a cube of 1,279 feet (almost the height of the Towers). Assuming a smoldering time of 18 hours as calculated above, each one-meter cube within the pile requires a total of 636 m³ of air in 18 hours, which is 1 m³ every 1.7 minutes. (Or more, of course, when allowing for the aluminum, glass, fireproofing, air conditioning plant, etc. It's also worth noting that, just to bring a single one-meter cube in the pile up to 600 °C, the entire air supply in 6,360 m³ or 6.82% of the pile would be required after allowing for the 0.1 porosity, and this isn't even allowing for the water and foam in the pores. Thus, all of the air contained in a 93,240 m³ pile could only bring 14 one-meter cubes up to 600 °C - even if the air was somehow capable of rapidly flowing through the pile, which it wasn't, and even if the water and foam wasn't there, which was not the case.)

A person who was buried alive in concrete dust or sand would clearly soon run out of oxygen. However, if the individual was being supplied with a cubic meter of fresh air every 1.7 minutes, they wouldn't even get a headache!

The official 9/11 conspiracy theory requires that people could quite happily be buried alive yards deep in concrete dust or sand (e.g. with some water bottles and rations, in a casket to relieve the pressure - but with holes drilled in top and bottom to let water trickle through) for weeks on end as millions of gallons of water percolated through the dust. Thus, this provides inspiration for an alternative to waterboarding that could be utilized on 9/11 suspects who were brought in for questioning. To find out what really happened on 9/11 within next to no time, they could be provided with a communications device in order to signal when they were ready to talk, and warned that if they subsequently lied or kept quiet, there would be no second chance...

Let's consider the possibility of natural 'chimneys' that were left in the pile and extended continuously up to the surface immediately after collapse, along with the more remote possibility of underground voids connecting the bottom of these vertical shafts with the PATH tunnels (although the north tunnel was completely filled to the west at the low midriver point). Air is drawn along from the subway and driven to the surface by buoyant convection. There is no evidence that any such continuous, open voids existed that could support the flow of air at any significant rate, but let's suppose for the moment that they did exist and there were some burning combustibles that just happened to have landed so that they were sticking out into this void. In reality, very little was left intact; office materials such as computers, telephones, chairs, tables and books were mostly pulverized into dust or powdered debris, along with the concrete. And only a tiny proportion of combustibles - less than 5% - was burning at the time of collapse.

A formula approximating the flow rate from stack effect in a chimney is:

Q = Cd×A×√(2×g×H×(Ti-Te)/Ti)

...where: Cd is a discharge coefficient generally taken as 0.62 to 0.7, A is the cross-sectional flow area of the chimney, g is the gravitational acceleration, H is the chimney height, and Ti and Te are interior and exterior temperatures respectively in Kelvin. In SI units of length, Q is in m³/s and g equals 9.802 m/s² (at New York City). Assuming Ti is 600 °C or 873 K and Te is 283 K, the flow rate for a chimney of cross-section 1 m² and height 21.3 meters works out at:

Q = 0.65 × 1 × √(2 × 9.802 × 21.3 × (873 - 283) / 873) = 10.9 cubic meters of air per second. At 1,200 g/m³ this is 13,100 g/s of air and 13,100 × 0.232 = 3,040 g/s of oxygen. At the 13.1 kJ/g-O₂ of complete combustion in a flaming fire, the potential heat release rate is 39.8 MW. For such a massive chimney, bits of fuel sticking out from the walls would burn up very quickly within minutes of the collapse - in the improbable event that any of the tiny fraction of fuel that was already burning at collapse time wasn't extinguished by the crushing, smothering, shredding, pulverization, scattering, etc. And any remaining smoldering as the fuel burned away into the walls would soon be extinguished by the hundreds of thousands of gallons of water that was hosed on the pile each day over the first ten days, with the dust washing over the surface and completely smothering the smoldering combustible.

The air flow rate is likely to be less, given that the tortuous path for incoming air at the bottom hardly resembles an open window or a vent in a building, and that the flow rate is related to the hydraulic diameter. The effective area determined by the hydraulic diameter can be much lower than the actual cross-sectional area. For example, a long narrow chimney might have been formed with its long sides bounded by steel columns. If there was a chimney of 1 inch by 10 feet by 70 feet deep, the hydraulic diameter D_H is a mere 2 inches, since D_H amounts to twice the width in the limiting case for a vent of width much smaller than its length. Although the basic formula for stack effect is not too far out with its prediction of a flow velocity of 10.9 m/s, its predicted flow rate is about 80 times too high. Using Cd = 0.65, g equals 9.802 m/s², H = 21.3 m, Ti = 873 K and Te = 283 K, the stack effect formula predicts a flow rate of 10.9 m/s times the cross-sectional flow area A. For a rectangular duct or tube of width 0.0254 m and length 1 m and up, the hydraulic diameter is approximately 0.05 m as per this online calculator. Say 0.0508 m.

Inputting the values outside temperature = 10 °C, inside temperature = 600 °C, height = 21.3 m, duct hydraulic diameter = 0.0508 m, duct length = 21.3 m and minor loss coefficient = 0, this online calculator predicts a duct velocity of 10.5 m/s and an air flow of 76 m³/h, which is 0.021 m³/s, i.e., 10.5 m/s × π × (½ × 0.0508⁾². Various alternative values of up to 1 for the minor loss coefficient bring about a minor reduction in the flow rate. So the 0.021 m³/s, at 1,200 g/m³, is 25.2 g/s-air and 5.85 g/s-O₂. Thus, at the 13.1 kJ/g-O₂ of flaming combustion, the potential heat release rate is 76.6 kW. Or if smoldering at 5 kJ/g-O₂, the maximum heat release rate is 29.2 kW. This compares with examples provided by FEMA, such as 100 kW for a medium wastebasket with milk cartons, or 17 kW for a crumpled double sheet newspaper, 22 g.

And the flow rate already assumes a temperature of 600 °C at the bottom of the chimney, which is unlikely given that only a few percent of the fuel was burning at collapse time. If the burning fuel was, say, halfway up the chimney, the height would be halved - the neutral plane is almost 3/4 of the way up instead of almost halfway up - and the air flow rate reduced by around 29.3%.

A "chimney fire" equivalent to a newspaper or a few milk cartons burning is not going to produce "molten streams" or a "little river" of any metal, and it isn't going to keep burning for months on end. It will burn out within minutes. NIST should have carried out some experiments to test their theory. For example, dig a channel in concrete dust or sand, throw in some aluminum sheets, steel beams that were certified to ASTM E119 by Universal Laboratories, pulverized carpet, paper, computer casings and milk cartons, try to ignite it, pour in plenty of water and fire retardant foam, and see if any metal melts over the following months. And see if people's rubber boots start melting when they walk over the top of the pile. Or NIST could have sponsored some tests on WTC-style floor assemblies, subjected them to greater heat exposure and protected them with less fireproofing compared to the WTC on 9/11, and observed whether or not the test specimens "were able to sustain the maximum design load" without collapsing for as long as the tests were run: 2 to 3 1/2 hours. Wait a minute! NIST did sponsor those floor assembly tests, and the results were inconsistent with the government's theory.

But the fact that the floor assemblies didn't collapse in NIST's tests is at least consistent with the fact that no collapses were observed in the Cardington fire tests of 1995 to 1997 that examined "the behaviour of a multi-storey steel framed building subjected to a fire attack", and found that no collapses occurred despite the columns being only "lightly protected up to a height of 20 mm below the connections", with the beams and the connections "totally exposed", and the "final test arrangement" being "over four times longer than the normal Standard fire resistance furnace" and "believed to be the largest gas fired test furnace ever constructed within a steel framed building". The main report, dated June 2000, for those Cardington tests appears to have provided NIST with the inspiration for its preposterous, unworkable 'theory' of the WTC7 collapse about "unrestrained thermal expansion" that "causes ends to move apart". And NIST had to ignore the report's conclusion, which stated that "The results from both, the Cardington experiments and the computer modelling of those experiments described in the previous chapters, demonstrate that the composite steel framed building tested exhibited inherently stable behaviour under the applied fire scenarios due to the highly redundant nature of the structural system." Neither did collapse occur in the 2003 Cardington test, in which unprotected steel was heated to 1,000 °C.

If there were any smoldering pieces of fuel burning away into the dust down in natural chimneys, then the rain and hose water would have flowed down into those chimneys, washing the dust along with it and smoothing over any holes in the walls leading to the fuel, smothering it. When someone digs a hole in the sand at the beach, the hole rapidly vanishes as soon as the first few waves flow over it when the tide comes in. The fire-fighters weren't fools; whenever they saw smoking holes in the pile they'd have directed the hoses at those holes. Thus, the smoldering fuel would be soaked and smothered, and would be extinguished.

Speculative scenarios of office combustibles burning in a void at the basement level and receiving air from the subway fail to work, because the fire zone in the Towers was towards the top of the buildings: around floor 93 and up for WTC1 and floor 79 and up for WTC2. Thus, any pieces of burning plastic or wood, etc., not only needed to avoid being extinguished by ten or more seconds of smothering / pile-driving / crushing / shredding / pulverization and scattering, but also needed to overtake 80 or 90 undamaged, non-burning floors beneath them on the way down (which would be especially ridiculous given that plastic, wood, paper, etc., are lighter than steel) to end up almost at the bottom of the pile, but not so low that they ended up in the bottom of the Bathtub that was flooded with 30 million gallons of water over ten days.

The old IRT Cortlandt Street/WTC station and subway, which was 40 feet below street level, sustained significant damage when the WTC collapsed. It was reportedly "located directly beneath the Twin Towers", and "the Towers essentially fell on the existing station". The following photos were released the week of September 24, 2001, and confirm that the subway was severely damaged by the collapses. Sections of the tunnels were blocked. None of the photos of these underground voids at the nycsubway.org link below shows the slightest evidence of any fire - no flames, and no smoke. Fires in that area would have rapidly depleted the oxygen and produced a lot of smoke and toxic fumes, making it too hazardous for photographers. Those who descended underground and reported seeing molten metal or molten steel - such as Leslie Robertson, William Langewiesche, Philip Ruvolo, and Richard Garlock - would not have been able to do so had raging fires of hydrocarbons burning in air been responsible for the molten metal. But their testimony is entirely consistent with molten iron produced by thermitic accelerants that contain their own supply of oxygen, with the ensuing extremely high temperatures possibly leading to a little smoldering and smoking of local hydrocarbons that are within voids containing sufficient air to sustain minutes of smoldering or seconds of flaming combustion.

IRT Cortlandt St/WTC station south end of northbound platform. Photo by MTA New York City Transit.

IRT 1/9 tunnel north of Cortlandt St. station. Photo by MTA New York City Transit.

IRT 1/9 tunnel looking north on southbound track at Liberty Street. Photo by MTA New York City Transit.

IRT 1/9 tunnel looking north on northbound track at Liberty Street. Photo by MTA New York City Transit.

Source: nycsubway.org

Any raging underground fires would have rapidly become starved of oxygen, and even if a few bits of wood or plastic were burning and sticking out into voids such as subway tunnels or chimneys that extended for twenty feet or so, they would not produce a "little river" of molten metal, neither would they partly evaporate steel. The interesting thing about these so-called "fires" is how the heat was consistently concentrated in the steel. For example, Larry Keating said, "Sometimes the steel could explode when the buried ends were exposed to the air. You saw some of the thickest steel I’ve ever seen bent like a pretzel, and you just couldn’t imagine the force that that took. The grapplers were pulling stuff out, big sections of iron that were literally on fire on the other end. They would hit the air and burst into flames, which was pretty spooky to see."

With conventional smoldering fires in a pile of debris, the burning debris would be the hottest materials, and any secondary heating of metal would necessarily be to a lower temperature. And in an oxygen starved fire, the smoke should have been dark. Instead, it was white, which is just how aluminum oxide (Al₂O₃) appears when dispersed into the atmosphere after fine aluminum particles are burned in the thermite reaction.

The driving force for stack effect is the pressure difference that results between lighter, warmer air and air of ambient temperature. A formula providing an approximation of this pressure difference is:

(P1 - P2) = C×A×H×(1/Te-1/Ti)

Wikipedia shows the pressure difference associated with a stack of given height as ΔP; it's shown here as (P1 - P2) to avoid confusion with the pressure gradient ΔP, the change per meter, used above for Darcy's law. C is a coefficient (0.0342 for SI units), A is the atmospheric pressure (Pa), H is the stack height (m), and Ti and Te are interior and exterior temperatures respectively in Kelvin.

Let's assume there is a hot, smoldering zone at 873 K, the exterior is at 283 K, the atmospheric pressure is 101,325 Pa, and the stack height is 21.3 m. So the pressure difference is:

0.0342 × 101,325 × 21.3 × (1/283 - 1/873) = 176 Pa. This is 8.27 Pa/m, compared to the 12 Pa/m normal difference with altitude for air of about 15 °C.

So not surprisingly, from q = -k × (P1 - P2) / (µ × L)

...where the fluid is air of dynamic viscosity 1.787×10^-5 Pa.s, a pressure difference of 176 Pa across a medium of permeability 1.71×10^-12 m² and length 21.3 m would induce a Darcy velocity of 7.91×10^-7 m/s, an air flux of 7.91×10^-7 m³/s per m² or 9.49×10^-4 g/m².s, and an oxidizer flux of 2.2×10^-4 g/m².s, more than three orders of magnitude below the 0.6 g/s required to sustain smoldering combustion.

Let's consider how much combustion could be supported by the air in typical voids within the pile just after collapse - the sort where fire-fighters could "get down below" and they'd "see molten steel, molten steel, running down the channel rails." Each void of 10 m³ would contain some 12 kg of air, with 23.2% of this initially being oxygen; hence, 2.784 kg of O₂. At first there would be sufficient oxygen to maintain flaming combustion (at 13.1 kJ/g of O₂ consumed). So 2.784 kg of oxygen could support the release of up to 36.5 MJ, although the fire would extinguish itself long before the oxygen had run out. This compares with an average fuel load in each 1 m x 1 m x 0.2 m compressed 'floor' of 8 psf or 3.63 kg per square foot or 39.06 kg/m² of wood equivalent fuel that could release some 10.78 MJ/kg in this compartment fire that would rapidly become fuel-rich and oxygen-depleted. There is a potential 421 MJ worth of fuel in an area of 1 x 1 m and a depth of 0.2 meters, or a potential 2.105 GJ within a one-meter cube, or a potential 21.05 GJ within 10 m³. So the potential 36.5 MJ from the oxygen within this 10 m³ void is a mere 1 / 577 of the 21.05 GJ of potential fuel. Or for smoldering combustion, the potential heat release at 5 kJ/g-O₂ is 13.92 MJ, which is 1 / 1,512 of the potential fuel. Various little bits of fuel - paper, plastic, etc., - that were sticking out into the void might burn under flaming combustion for minutes or smolder for hours, but they certainly wouldn't be capable of melting metal for weeks or months on end.

So, any burning material that was totally smothered by the concrete dust would simply be extinguished immediately. Where chimneys reached the surface, there might have been some flaming combustion for a matter of minutes and smoldering combustion for hours. However, as the smoldering combustibles at the chimney boundary burned out, the situation would revert to that of cold or cooling combustibles that were smothered by tens of thousands of tons of concrete dust, even if the fuel had improbably escaped being soaked by some of the millions of gallons of water that percolated down through the dust and along any chimneys, or being coated with fire retardant foam that had been diluted to increase its range, or being completely smothered by dust that the water washed over it. Much of the heat would be vented out to drive the smoke plume, and remaining energy would be conducted away through the concrete and massive steel members, with the mean debris pile never being more than 10 °C above ambient temperatures (very briefly, immediately after collapse) and no more than 3 °C above ambient for most of the time, if the government account were true. (Those figures of course refer to the 208' x 208' footprint of a Tower. The area of the Bathtub, at 980' x 510', was nearly six times greater than the combined WTC1 and 2 footprints.)

It is known that underground coal fires can reach high temperatures, e.g. Australia's Burning Mountain reportedly reaches 1700 °C deep underground. The operative word here is coal. And these fires self-extinguish when the overburden becomes too thick and fractures fail to reach the surface to draw in more air. The 1700 °C quoted in popular reports is rather high compared to underground coal fire temperatures stated in the literature, e.g. "up to 1200 °C". Glenn B. Stracher's Geology of Coal Fires states temperatures of 550-1450 °C for coal fires, 550-1650 °C for bitumen fires, and 550-2400 °C for oil fires.

However, underground fires of coal, bitumen or oil are all most unlike the WTC debris pile, which was by mass about 97% non-combustibles such as tightly compacted concrete dust, steel columns, floor decking, air conditioning and refrigeration plant, aluminum, glass, and fireproofing. Note how the potential fire temperature, e.g. up to 2400° C for oil, is strongly correlated with the heat of combustion. As you move up from lignite through to bituminous coal to oil, the heat of combustion - and the adiabatic flame temperature - increases. Coal yields up to 35 MJ/kg, whereas the WTC debris pile would struggle to average 1 MJ/kg.

Crucially, even underground coal fires require an oxygen source, and there was no such source within the WTC debris pile. Stracher's Geology of Coal Fires has this to say about how the fires are aerated:

The two necessary components for combustion are fuel and oxygen. Rugged relief and steeply dipping fuel-bearing strata provide ideal conditions for natural coal bed fires. Coal beds usually are ignited in the steep walls of river valleys and on steep hillsides that are not protected by Quaternary sedimentation. Fuel-bearing layers that are shielded by alluvial sediments commonly are not ignited (Burg et al., 1991, 1999). When fuel-bearing strata burn, a flame front develops along steeply dipping beds, and the fire descends quickly to depth. Fires occur mostly in beds that dip from 30° up to subvertical (see Table 1). A unique example of a fire spreading within near-horizontal strata is in the Powder River Basin, Wyoming, United States (Heffern and Coates, 2004). When a fire starts, it first spreads along an outcrop. With time, it burns deeper into the hillside and causes the overlying rocks to subside progressively into the burned-out void. The resulting fracturing allows air to enter and gas to escape, so that the fire continues (Fig. 1). These fires extinguish themselves naturally at the point when the overburden becomes so thick that fractures from the collapse fail to reach the surface to draw in more air, or when the fire burns down to the water table in the coal.

(In the above, the third requirement for combustion - heat - could have been included, along with fuel and oxygen.)

And another hint is provided by this source:

The rubble zone and the fault closest to the burning coal front are the main path ways to a deep coal fire, as these highly permeable zones act respectively as an air intake and exhaust under practical heterogeneous permeability conditions.

So, there are the questions: Was the WTC debris pile on a mountain or a steep hillside? Was most of the WTC pile comprised of a fuel, e.g. coal or oil, as opposed to concrete dust, fireproofing, steel beams, glass, etc? Did the WTC pile have fuel bearing strata? Was potential fuel in the pile not shielded by the equivalent of alluvial sediment, e.g., tens of thousands of tons of fine-grained concrete dust that had been deposited on top of it? Was there subsidence of overlying rock resulting in fracturing and exchange of gases so that the fires could continue? Was it unlikely that the overburden was so thick that any fractures or chimneys would fail to reach the surface to draw in more air and the fires would consequently extinguish themselves naturally? Did the pile have suitable channels that could have acted efficiently as air intake and exhaust?

If your answers are mostly no, then the answer to the following will also be "no".

Were conditions in the heavily watered, foamed and smothered WTC pile suitable for an underground fire that continued to burn for months on end and was like a "foundry" and like "lava" with "molten steel running down the channel rails"?

Finally, we have enough information to describe the reality of the 'fires' at Ground Zero. Early into the collapses, most or all of the tiny fraction of fuel burning at collapse time is extinguished by the supposed "pile-driver" effect of the collapse as the office combustibles are shredded, pulverized and smothered whilst crushed under the weight of tens of thousands of tons of upper floors for ten seconds or more. (This compares with a 960 kW fire in a 1 m³ void - not totally smothered! - that takes 3.79 seconds to consume all of the oxygen.) The maximum possible temperature increase from friction during the collapse - if all the energy is converted to heat and remains within the material - is given by gh/c where g = 9.802 m/s² (the gravitational acceleration constant for New York City), h = 1,368 / 3.2808 m (the maximum height over which the collapse occurs), c (specific heat) = 450 J/kg.K for steel (at around ambient temperature) and c = 800 J/kg.K for concrete, which leads to 9.08 °C for the steel and 5.11 °C for the concrete.

Immediately after collapse, the system potentially starts with combustion at several remaining points along the x, y and z axes, albeit these points comprise no more than ~0.5% of the total volume - even if none of the fire was extinguished by the collapse. At points that are completely smothered, any remaining fires are unequivocally extinguished. Even smoldering cannot be sustained, since the oxidizer flux is at least 1,775 times below the minimum requirement of 0.6 g/m².s-O₂.

In the case of natural chimneys that happen to reach the surface of the pile, any intact combustibles that improbably happen to be burning and half sticking out into the chimney burn very well at first, but quickly burn away into the concrete dust. Over the first ten days, the bottom ten feet or so of the Bathtub (the actual height extends at least to the level at which it is being pumped out at Jersey City, as the north PATH tunnel is "completely filled" at the low midriver point) is flooded by 26 million gallons that leaks from the Hudson River and from broken mains, together with 1 million gallons of rain and 3 million gallons hosed onto the pile by fire-fighters. Any burning material that falls down to the bottom is immediately extinguished. The Bathtub is 980 ft x 510 ft and 3 million gallons of water has a volume of 401,042 ft³, so 3 million gallons per day per day makes for a height of 401,042 / (980 × 510) = 0.802 feet per day, which after allowing for 0.1 porosity corresponds to 100% saturation over a height of ten feet in barely more than a day.

Fire-fighters direct their hoses at any smoking holes in the ground, and so these chimneys certainly get their fair share of the four million gallons of water that flows right down through the pile from top to bottom within the first ten days, along with thousands of gallons of Pyrocool FEF fire retardant foam. Above the water level, the running water from the hoses washes the dust along until it covers any holes in the chimney walls leading to any smoldering plastic, paper, computers, wood, etc., totally smothering the sodden materials and extinguishing any remaining vestiges of smoldering.

Within voids that are formed in the pile, e.g., underneath massive columns, the fuel can initially burn in flaming combustion (but only for a few seconds), and thereafter a minuscule proportion of the fuel might continue to smolder. Since only ~5% of the fuel is burning at the time of collapse, and much, if not all, of that would have been extinguished by collapse, and the fuel is less than 10% of the volume, the most likely scenario is that none of the fuel that happens to settle at the bottom of a void is burning at collapse time, any fuel that is burning is completely smothered elsewhere in the dust and thereby extinguished, and thermitic material that should not have been present is responsible for all subsequent generation of heat, including possible temporary ignition of office combustibles.

Let's cut to the chase. The mass flux of oxidizer through the debris is calculated above at 0.000338 g/m².s-O₂, which at 5 kJ/g-O₂ for smoldering combustion can support a heat release rate of 1.69 W/m². It doesn't matter whether the fire load is 20 MJ or 200 MJ of fuel per m². The consumption of oxygen within the void cannot exceed the supply of oxygen for any longer than it takes for oxygen levels to drop too low to sustain smoldering. The  oxygen originally within the void provides some reserve capacity, but only for a matter of minutes. 1.69 W/m² is barely more than 1/1,000th the level of overhead sunshine, and wouldn't be enough to warm your little finger. It's equivalent to a third of a burning cigarette per square meter. It obviously cannot translate into "a noticeable difference between the temperature in the middle of the site" above thousands of tons of concrete dust and steel compared to "when you walked two blocks over on Broadway"; heat that "you could actually feel". In January, that is, four months after the collapses!! And it isn't going to melt any metal - whether steel, aluminum, tin or lead - or heat steel to cherry-red (about 750 to 900 °C) in six or seven weeks. (We'll confirm this in a moment!)

In theory, smoldering could be maintained within a void for some time, but the long-term power output or heat release rate of the fire could never exceed 1.69 W/m². For example, if pulverized fuel is lying on the floor of a 2 m x 1 m x 0.5 m high void, mixed with concrete dust, taking up one-tenth of the area and all smoldering at a typical rate of 6.5 kW/m², the total heat release rate is 2 × 0.1 × 6,500 = 1.3 kW. Given the void volume of 1 m³, the air within initially has a density ~1.2 kg/m³, so multiplying 1.2 kg by 23.2% oxygen by mass we have 278 g of O₂, which at the 5 kJ/g-O₂ of smoldering combustion can release 1.39 MJ. A consumption rate of 1.3 kW would use up 1.39 MJ worth of oxygen in 1,069 seconds = 17.8 minutes. The 1.3 kW over 2 m² is 650 W/m². Thus, smoldering can only be sustained if on average no more than 1.69 / 650 = 1/385th of the fuel front (by surface area directly facing the oxidizer) is smoldering.

In this example of a void with a 2 m² floor area and fuel taking up 10% of this area, smoldering can only be sustained in 0.2 m² / 385 = 5.2 cm² of fuel. If a typical piece of fuel is a 1 mm cube and they're all neatly laid out one deep, then there would be 200,000 cubes of fuel, but the oxygen supply could only support the smoldering of 520 of these pieces (at any one time). Over 1 m², half the floor area, the 260 one mm by one mm fuel fronts totals 0.00026 m² of fuel per m², so if this fuel has a heat release rate at its smolder front of 6.5 kW/m², the square meter area of the void has a total heat release rate of 0.00026 × 6,500 = 1.69 W. Or with each little cube having an output of 6.5 mW, with 260 of them smoldering per m² of void floor, the total output is 1.69 W/m².

In the general case of fuel pieces in various shapes and sizes scattered randomly, the maximum proportion of fuel front that can burn (dimensionless) equals the heat release rate that can be supported by the oxidizer flux (e.g. W/m² which is derived from g/m².s of O₂ times heat released in J per g of O₂) times the floor area (m²) divided by the mean burning rate of the fuel (W/m²) and the area of the fuel (m²). But we don't need to know the details of the fuel in order to disprove the government's conspiracy theory. It's the low oxygen supply rate of 0.000338 g/m².s that dictates the low maximum possible heat release rate of 1.69 W/m² from fuel that requires an oxidizer, as opposed to accelerants - e.g. thermite - that contain their own oxidizer.

The point about fuel is made because in order to get the government's 'fires' theory to work just to the point of producing 1.69 W/m², it is necessary for exactly the right proportion of the fuel to be smoldering. If it's too low, the heat release rate doesn't even get up to 1.69 W/m². If it's too high, the oxygen in the void is exhausted, the smoldering fuel is extinguished, and the air refresh rate is so low that the fuel cools down and has no hope of re-igniting by the time oxygen levels have been replenished.

Under flaming combustion the air in the void is consumed in seconds. In the example above, the oxygen is fully consumed in 17.8 minutes when all the fuel is smoldering. But combustion will cease before the oxygen gets to zero. The "reserve capacity" is less than ten minutes, although it does mean that within this air pocket (as opposed to a region that's completely smothered by concrete dust) there is time for the air to diffuse to the fuel so that smoldering can be sustained - and that's only provided the oxygen consumption is sufficiently low, and until the fuel burns out. If the oxygen gets used up, the refresh rate is 0.000338 g/m².s, and so in this example where we have a floor area of 2 m² and a height of only 0.5 m, it takes 278 / (2 × 0.000338) = 411,243 seconds = 4.76 days to replenish the 278 g of oxygen from the original 1 m³ of air. Or if combustion stops when the oxygen drops by half, it takes 11.4 hours just to return to 60%, by which time the fuel has also cooled down.

Moreover, the oxygen is only sufficient to allow smoldering of the tiny fraction of fuel, e.g. 1/385th, at one particular height (z co-ordinate) - the first (highest) level at which it encounters smoldering fuel as it permeates down into the pile. The air cannot "rejuvenate" itself to go on and oxidize any of the fuel at lower levels. Or alternatively, if less than the calculated proportion, e.g. 1 in 385, of the fuel at some particular level (for some particular area in the horizontal plane) is smoldering, there would be some remaining oxygen for additional fuel above or below.

For example, there could be a 1.69 W/m² void with smoldering fuel at the (x, y, z) co-ordinates (10, 20, 10) that extended possibly for several meters horizontally, and another higher up at (15, 25, 15). But a void at (15, 25, 10) could not support smoldering, since the oxygen would have already been used up by smoldering in (15, 25, 15) above.

Horizontal diffusion of the incoming air should have been easily capable of sustaining such low-level combustion within a void, in contrast to the fact that no smoldering could be sustained in the dust. The 1.69 W/m² is 1,775 times short of the minimum smolder power density of 3 kW/m², or the oxidizer flux at 0.000338 g/m².s-O₂ is 1,775 times short of the minimum 0.6 g/m².s-O₂. And horizontal air permeation through the dust would similarly be too low. In a void, the oxygen has plenty of time to diffuse to the smolder fronts. If the fire's power output is only 1.69 W/m², then oxygen consumption equals the oxygen supply. The Darcy velocity of the incoming air is predicted to be 1.169×10^-6 m/s, and the actual velocity through the 0.1 porosity is 1.169×10^-5 m/s. If the void is a one meter cube, the air initially had a reserve capacity of 1,200 g × 0.232 × 5,000 J/g / 1.69 W = 823,669 seconds = 9.53 days at this consumption rate.

As for venting away the exhaust gases, that is more likely to pose a problem for the 'fires'. The exhaust would probably have to diffuse sideways, which would inhibit smoldering in adjacent voids, but these could possibly be at some other height (z co-ordinate). With a Darcy velocity of 1.169×10^-6 m/s and ten times that for the actual velocity allowing for the 0.1 porosity, if the exhaust gases permeate through at a comparable velocity, they would take 23.8 hours to ascend one meter, so would surely cool and stop ascending well before clearing the pile. After all, NIST's Dr. Ohlemiller describes a reverse smolder front of a few kW/m² from a source 0.1 to 0.15 m in diameter as so "weak" that the buoyant plume might not even reach the ceiling of a room. The plume from a 1.69 W/m² source wouldn't manage to ascend through yards and tons of concrete dust!

The probability that voids of smoldering fuel could encompass the entire 4,019 m² of footprint per Tower (and all of Building 7's), and all of them would have exactly the right proportion of fuel smoldering, and the fuel in each void could somehow maintain smoldering for five months, or there could be a bizarre series of heat conductions from cool 1.69 W/m² voids through concrete dust to ignite fuel in even colder voids elsewhere such that the output was maintained across the entire 4,019 m² for five months, may be safely stated as zero.

Even under this zero probability scenario, the maximum sustainable heat release rate of the 'fires' within a collapsed Tower footprint of 4,019 m² could be 1.69 W/m², which is a total of 6.792 kW. Let us suppose that the impossible occurs: that this is in fact sustained for a full five months, and is maintained over the entire 4,019 m² of the footprint, and that all of the heat remains in the pile (despite photographic evidence to the contrary, e.g. white smoke). So over five months (September - February), the total heat released is 153 × 24 × 3,600 × 6,792 = 89.8 GJ. There was 90,000 tonnes of steel per Tower. Firstly, let's suppose that the entire 89.8 GJ is used to heat the steel, and forget about the concrete. Pretend it's self-heating or something. Or pretend it's just a bizarre coincidence that the heat is somehow mysteriously concentrated in the steel - just as if it had been targeted with thermite.

In order to raise the temperature of 90,000 tonnes of steel by 1°C, the energy required is:

90,000,000 kg × 450 J/kg.K = 40.5 GJ.

Thus, the entire heat output from the 'fires' in the pile, if locked in for five months, could increase the temperature of the steel by 89.8 / 40.5 = 2.2 °C. This is hardly going to turn it cherry-red!

Now we include the heat required to raise 110,000 tonnes of concrete by 1°C.

110,000,000 kg × 800 J/kg.K = 88 GJ.

Thus, the entire heat output from the 'fires' in the pile, if trapped in for five months, could increase the temperature of both the steel and the concrete by 89.8 / (40.5 + 88) = 0.7 °C.

This is barely more than 1 Fahrenheit degree.

And the (im)possibility of the (non-existent) 89.8 GJ over five months and 4,019 m² of footprint scales down to a (non-existent) 22.3 MJ per m² over 153 days, with the mass of steel, concrete, aluminum, etc., on average scaling down in a similar proportion. In reality, a few smoldering pieces of burning plastic or wood in an oxygen-starved underground void are going to extinguish within minutes or hours. A 1.69 W/m² 'fire' is equivalent to one burning cigarette every three square meters, or a burning candle every 47.3 square meters - e.g., a single candle in a 22.6 feet by 22.6 feet room.

Even if we include the unlikely "chimney fires" as described earlier, a chimney of hydraulic diameter two inches, with a potential heat release rate under flaming combustion of 76.6 kW equivalent to a few milk cartons, is going to burn out within minutes. According to Babrauskas, a 6.6 liter waste basket of empty milk cartons can produce a peak heat release rate of 70 kW, and the time of intense burning (at over 50 kW) is 200 seconds. So the total heat released will be ~14 MJ. Thus, it would take 89,800 / 14 = 6,414 of these highly improbable chimney fires per Tower footprint - in which any burning fuel prior to collapse wasn't extinguished by more than ten seconds of crushing and smothering - just to match the 0.7 degree increase of the 1.69 W/m² 'fires'.

We could include the maximum possible temperature increases of 9.08 °C for the steel and 5.11 °C for the concrete from friction during the collapse, although in this case we have to pretend that the entire gravitational energy was used to heat the steel and the concrete, and pretend that none of it was converted to sound, or used to eject massive steel beams laterally, pulverize the concrete, and smash the intact, cold, non-burning lower floors, including the breaking of hundreds of steel connections per second. And even then, no metal is going to melt.

A 1.69 W/m² 'fire' is most definitely not going to melt any metal. Period.

The official theory has to clear an impossible series of hurdles even before it can achieve the above maximum possible temperature increase in the pile of 0.7 °C, which is before we allow for the heat vented out with the smoke and the requirement to heat the 43,600 aluminum curtain wall panels of around 100 pounds, the glass, 56 tons of fireproofing per floor, 200,000 pounds of the nonflammable, noncorrosive, nontoxic refrigerant Freon 22, etc. For example, early into the collapses of the Towers, the upper floors (where the fires were located) were being crushed as if by a pile-driver, according to Ronald Hamburger, a contributor to FEMA and NIST. Hamburger had previously said (before learning that a cover-up was required), "It appeared to me like charges had been placed in the building."

Fuel burns much faster under flaming combustion compared to the typical 6.5 kW/m² of smoldering. According to FEMA, for example, "the associated energy release rate per unit surface area [in typical office building fires] ranges from 320 to 640 kW/m²".

Let's take 480 kW/m², and assume 13.1 kJ/g of O₂ consumed. A 1 m³ void contains 1.2 kg of air including 278 g of oxygen. Thus, if we have a void of 2 m x 1 m x 0.5 m deep as in the example above and fuel is burning in flaming combustion at a heat release rate of 480 kW/m², the 960 kW over 2 m² consumes oxygen at a rate of 73.3 g/s and the oxygen is fully consumed in 3.79 seconds. Even taking a minimum estimate for the collapse time of ten seconds, it is not very credible that materials burning at 480 kW/m² could have been crushed, shredded, pulverized, scattered, and could remain burning for ten seconds or more in the absence of oxygen whilst crushed under the weight of tens of thousands of tons of upper floors, going on to end up protected in a void in which they could burn for another few seconds. After all, when some drunken lout ignites newspapers and places them on several seats on a bus, the fires are all extinguished when the driver stamps on them a few times. And people with burning clothes - even after being sprayed with oil and gasoline - can roll over to extinguish the fire.

Given that just about everything apart from huge, twisted pieces of steel - computers, telephones, chairs, tables, filing cabinets, books and gypsum along with the concrete - was pulverized into dust or powdered debris, it makes most sense to consider the various combustibles as being of a similar size to the concrete dust. If the distribution of sizes is similar for the fuel and the concrete dust, then there are 30% within the range 10 cm to 1 cm, 20% within 1 cm to 1 mm, 15% within 1 mm to 100 µm, 10% within 100 µm to 10 µm, and 25% at less than 10 µm.

The speed at which the smoldering fire front advances is given by the power density divided by the heat of combustion and the density of the fuel. So kW/m² / [(kJ/kg) × (kg/m³)] ===> m/s. In the smoldering case of 6.5 kW/m², assuming 21.56 MJ/kg and 200 kg/m³ we have:

6.5 / (21,560 × 200) = 1.51×10^-6 m/s = 5.43 mm/hour = 97.7 mm in the 18 hours typical time for the pocket of fuel to be consumed. So a fuel density of 200 kg/m³ and maximum size of 10 cm fits in with the ~18 hours smoldering time assumed above. Some fuel would have a lower density and so would burn up in less time (for the same maximum size). 6 pcf or 96 kg/m³ is regarded as a "high" density for polyurethane foam, for example. Combustible material compressed within the concrete dust would have a higher density, but combustion there is precluded by total smothering.

Or in the flaming combustion case of 351 kW/m², the velocity of the fire front is 8.14×10^-5 m/s, 54 times faster, again at 97.7 mm, in this case over the 20 minutes typical time for the fuel to be consumed.

Let's consider a 1 mm cube as a typical size. The volume is 10^-9 m³, and assuming 200 kg/m³, the mass is 2×10^-7 kg.  The 1 mm cube has a smolder front area of 10^-6 m² and a heat release rate of 0.0065 W. The average heat of combustion of the fuel is taken as 21.56 MJ/kg, and so the combustion of one of these cubes will release 4.312 J. Thus, the 4.312 J would be released over 4.312 / 0.0065 = 663 seconds which is ~11 minutes. Dividing 5 months into 11 minutes, there would have to be a series of 20,029 smolderings and transfer of heat to ignite the next piece of fuel.

At a smoldering rate of 5.43 mm/hr, a 3 cm cube of fuel burns up in 5.52 hours, a 3 mm cube in 33.1 minutes, 300 µm in 3.31 minutes, 30 µm in 19.9 seconds, and 3 µm in 1.99 seconds. Pieces of 15 µm and smaller would have burned out before the Towers' fire floors even reached the ground. Even a 1,000 kg/m³ fuel with a length of 10 cm and smolder velocity of 1 mm/hr, say, would burn up within 100 hours or ~4 days.

If too much fuel is burning, oxygen levels would be expected to drop too rapidly to allow a few pieces of fuel to remain smoldering. Even if a sustainable proportion of fuel was smoldering, the 1.69 W/m² within the void is orders of magnitude below the kilowatts/m² required to ignite smoldering combustion, and even further short of the 20 kW/m² required for piloted ignition of a a range of common combustibles. At 1.69 W/m² and 21.56 kJ/g, the mass burning rate is 1.69 / 21,560 = 0.0000784 g/m².s. Under equilibrium conditions in which the smoke doesn't crowd out the oxygen, the concentration of smoke would be very low and the upper gas layer, marginally less cold than the incoming air, would be very thin. Gas temperatures would be well below the autoignition temperature of any available fuel. If the fire burns more rapidly and smoke generation exceeds the supply of fresh oxygen, the fire extinguishes.

And in order to sustain the less than lukewarm 1.69 W/m² for months, the government conspiracy theory requires a vastly improbable scenario of adjacent voids containing fuel, with an extraordinary series of conduction of heat through the intervening concrete dust and subsequent ignition of fuel in the next void. Moreover, since there were a number of hot spots in the pile at any given time, the official theory requires that there are many of these most improbable series running in parallel, for months on end. The problem is that the smoldering voids generate very little heat in the first place, but even if they were hot enough, insufficient heat would be conducted across the concrete to the adjacent void before the original void had burned out and started cooling.

If there is a hot spot at a particular point in the pile, the speed at which heat is conducted through the pile is related to the thermal diffusivity of the conducting material. The time t required to heat up a point at a distance x to a temperature midway between the temperatures of the hot spot and ambient temperature, is related to the square of the distance. Conversely, the distance x is related to the square root of the time t.

The concrete or steel is treated as a semi-infinite solid or bar. If a bar of semi-infinite length with insulated sides is "instantly" heated at one end, the distance x at which half the temperature rise occurs is given by:

x = 2 × z × √(α × t)

where z is the value required to yield a Gauss error function of 0.5, t is the time in seconds, and α is the thermal diffusivity of the material in m²/s. Since erf(0.477) is close to 0.5, z is taken as 0.477. Thermal diffusivity (α) is the thermal conductivity (k) divided by the volumetric specific heat capacity (ρ × C), where ρ is the density and C is the specific heat by mass. It is also useful to find the time t, given the distance x.

So rearranging x = 0.954 × √(α × t), we have near enough to:

t = 1.1 × x² / α

(See this page for example, where their Eq. 4.3 can be used to derive those equations for solving x and t.)

This underestimates the time required for a given distance, because the sides of the "bar" are not insulated. Heat is being conducted in other directions, except in the case of a massive wall of fire, in which case it would be relatively accurate at the center. At Ground Zero, there should have been no great walls of raging fire.

The thermal conductivity k for lightweight concrete and normal weight concrete is 0.1 to 0.3 W/m.K and 0.4 to 0.7 W/m.K respectively. Assuming the concrete dust is packed so as to be closer to the original density of lightweight than normal weight, we'll take the density as 1,750 kg/m³, and the thermal conductivity as 0.3 W/m.K.

So for the concrete, we have:

α = 0.3 W/m.K / [1,750 kg/m³ × 800 J/kg.K] = 2.14×10^-7 m²/s

And for steel:

α = 40 W/m.K / [7,860 kg/m³ × 550 J/kg.K] = 9.25×10^-6 m²/s

So for concrete that is 610 °C at the hot end and an ambient temperature of 10 °C, the time taken for a point 1 meter distant to reach 310 °C is 1.1 × 1² / 2.14×10^-7 = 5,140,187 seconds = 59.5 days. Or for a point 40 mm distant, we have 1.1 × 0.04² / 2.14×10^-7 = 8224 seconds = 2.284 hours. Or the time for a point 10 mm distant to reach 310 °C is 1.1 × 0.01² / 2.14×10^-7 = 514 seconds = 8.57 minutes. The time associated with one meter is way beyond the time that any smoldering combustibles within a void would take to burn up. A wall of concrete dust that was only 40 mm thick would have collapsed.

For steel, the corresponding times are shorter, or the distances are greater, as one would expect from its higher thermal diffusivity. The time taken for a point 1 meter distant to reach 310 °C is 1.1 × 1² / 9.25×10^-6 = 118,919 seconds = 33 hours. Or for a point 0.5 m distant, the time is 1.1 × 0.5² / 9.25×10^-6 = 29,730 seconds = 8.26 hours.

From x = 2 × z × √(α × t)

...we can find the distances associated with typical smoldering times, say, 18 hours. So for the concrete, x = 0.954 × √(2.14×10^-7 × 64,800) = 0.112 m. However, the 1 mm fuel cube burns up in 663 seconds (11.05 minutes). This is associated with a distance of 0.954 × √(2.14×10^-7 × 663) = 11.4 mm = 0.45 inch.

Or for the steel at t = 11.05 minutes, x = 0.954 × √(9.25×10^-6 × 663) = 74.7 mm = 2.94 inches. At 18 hours, x = 0.738 m.

(For those who are curious as to how the above formulae compare with a rough approach that estimates the heat conduction rate and the energy required to heat the material, and divides the latter by the former to obtain the time, the standard one-dimensional equation for the quantity of heat conducted per unit time is:

q = -k × A × (T1 - T2) / x

...where q is in J/s or W, k is the thermal conductivity in W/m.K, A is the area of the conducting material in m², T1 and T2 are the temperatures at the ends of the conductor in °C or K and x is the thickness of the conductor. So (T1 - T2) / x gives the thermal gradient in K/m. The minus sign in k just denotes the direction of heat flow.

Then there is the energy required to raise the mass of material by the required temperature:

E = A × L × ρ × C × (T1 - T2)

...where A is the area of the "bar", L is its length, ρ is its density, and C is its specific heat. If the cold end goes up from ambient to the mean of ambient and the hot side, the average temperature difference could be taken as three-quarters of the difference between hot and ambient for purposes of calculating q. Recalculating the difference every second is more accurate, and adds about 4% to the predicted time for a particular distance. And on average the temperature of the material is increased by three-quarters of the difference between hot and ambient. This also underestimates the time taken, since it does not allow for the fact that the material must also be heated at distances beyond x.

Since the time required is the energy E divided by the rate of heat flow q through the conductor, and L and x are the same, we have:

t = A × L × ρ × C × (T1 - T2) / [k × A × (T1 - T2) / x]

= L × ρ × C  / [k  / x] = x² × ρ × C  / k

Since ρ × C  / k = 1 / α, we have:

t = x² / α

Cf. t = 1.1 × x² / α

...which has an extra factor of 1.1, making some allowance for heating the material at a distance greater than x.)

So, if the impossible occurs and a few pieces of smoldering fuel heat the hot end of the concrete to 610 °C, then the government conspiracy theory requires a further series of impossibilities in raising the temperature of the cold end of the concrete from 10 °C up to 310 °C in order to reach the auto-ignition temperature of some - but by no means all - potential fuels. The thermal diffusivity of the concrete is too low to reach adjacent pockets of fuel. And 'fires' equivalent to one-third of a burning cigarette per m² will not be capable of raising the temperature of structural steel members or hundreds of pound of concrete by any appreciable amount.

A forensic pathologist can estimate the time of death from the temperature of the corpse, since the cooling rate ranges from 0.8 to 1.5 °C per hour. Similarly, the condition of the WTC steel provides information as to whether or not accelerants were used to bring down the buildings. Steel from the WTC was found to be cherry-red (about 750 to 900 °C) six or seven weeks after the collapses, in the last week of October 2001.

The most massive steel columns in floors encompassing the fire zone of WTC1 were the core columns at the corner of the core, such as column 501 and 1008. These were W14x730 wide flange shapes around floors 83 to 86 (NIST Appendix E, Fig. E-6), and in the fire zone such as floor 98 were most likely of size W14x257 (as implied by NIST-published data on demand : capacity ratios and the gravity load). The mass of these columns is 257 lb per foot. The flange thickness is 1.89 inches; web thickness is 1.175 inches; the distance from one outside edge of a flange to the outside edge of the opposite flange is 16.38 inches, and the breadth of the flanges is 15.995 inches. The perimeter of the column, known as the "heated perimeter" for heat transfer calculations, is 94.39 inches. However, heat being lost from the inside of the flanges will be met by heat from the other flange and from the web. So let's be generous and suppose that heat is only being lost from a heated perimeter of 2(bf+d), which is twice the breadth of the flanges plus twice the depth between the two outside flange edges. This makes it 64.75 inches.

Let's suppose that the office fires somehow managed to heat this massive steel column to cherry-red - in spite of the fact that they couldn't have, and let's suppose that corresponds to a temperature of 750 °C. Each meter-length of the column has an area of 1 m x 64.75 / 39.37 = 1.64 m² through which it loses heat to the concrete dust by conduction. (Heat loss by radiative transfer would be greater. And for simplicity we ignore heat conducted diagonally from the steel column, and from each end, which favors the government account.) Over a period of six weeks, the formula for thermal diffusivity as used above will indicate how much concrete would be heated - provided there was enough enthalpy in the steel to supply the required heat to the concrete.

x = 0.954 × √(α × t)

α for concrete is 2.14×10^-7 m²/s, and t for six weeks is 3,628,800 seconds. Thus, x = 0.841 meters, the distance at which half the temperature increase occurs at time t.

Along four cross-sections of concrete of total area 1.64 m² and length 0.841 meters, the hot end is at 750 °C, and the cold end goes from 10 °C to 1/2 (10 + 750) which is 380 °C over six weeks. The average temperature of the cold end is approximately 1/2 (10 + 380) = 195 °C, which we shall take as T2.

From q = -k × A × (T1 - T2) / x

...we have q = 0.3 W/m.K × 1.64 m² × (750 - 195) / 0.841 m = 325 W. This area of 1.64 m² corresponds to a 1 meter length of the steel. Each foot weighs 257 lb, so a 1 m length has a mass of 257 ×3.2808 / 2.2046 = 382 kg. Taking the heat capacity of the steel as 550 J/kg.K (allowing for the higher temperature), a loss of 550 × 382 = 210 kJ corresponds to a 1 °C reduction in the steel temperature. A loss of enthalpy at a rate of 325 W for six weeks equates to a total loss of 1.18 GJ. So 1,180,000,000 / 210,000 = 5,619 °C.

Thus, the energy required to raise the concrete to temperatures ranging from 750 °C at the point in contact with the steel to 380 °C at a distance of 0.841 meters after six weeks, i.e., 1.18 GJ, greatly exceeds the energy that was bound up in the steel to raise it from 10 °C to 750 °C, i.e. 740 °C × 382 kg × 550 J/kg.k = 155 MJ. Long before the six weeks was up, the steel would have cooled to much closer to ambient temperature. There is zero chance of it remaining cherry-red for six weeks if the government account were true.

A smaller column such as a W14x53 would have cooled in even less time. If we again count 2(bf+d) as the heated perimeter (it should really be more to allow for heating the two U-shaped areas of concrete), then that gives 43.96 inches. So 53 lbs per foot is 78.87 kg per meter length, and a heated perimeter of 43.96 / 39.37 m x 1 m length is an area of 1.12 m² in contact with the concrete.

So with this column we have conduction into the concrete q = 0.3 W/m.K × 1.12 m² × 555 / 0.841 m = 222 W. A 1 °C reduction in steel temperature corresponds to a loss of 550 × 78.87 = 43.4 kJ, and a 740 °C reduction in steel temperature corresponds to a loss of 32.1 MJ, which at a rate of 222 W would occur in 144,595 seconds = 40.2 hours. Of course, the steel would be quickly cooling down, lowering the thermal gradient, resulting in less conduction through the concrete and a time of greater than 40.2 hours to cool towards ambient temperature. However, the thermal gradient through the concrete is initially greater, with the 380 °C point in the concrete then being much closer than 0.841 m from the steel.

And the "Arabs, office fires and a run of bad luck and bizarre coincidences did it all" conspiracy theorist has already lost the argument just as soon as the steel has cooled to below cherry-red temperatures.

Going back to the W14x257, in order for this to cool from 750 °C to 500 °C, which would take it down to "black red/red-gray" or "faint red", it would need to lose 250 × 550 × 382 = 52.5 MJ. Assuming the steel temperature averages at 625 °C and a point 0.045 m along through the concrete goes from 10 °C to 318 °C, say, averaging about 164 °C, the rate of heat flow through the concrete is:

q = 0.3 W/m.K × 1.64 m² × (625 - 164 °C) / 0.045 m = 5.04 kW. So 52.5 MJ / 5.04 kW gives a time of 10,417 seconds = 2.893 hours. A check with the formula:

x = 0.954 × z × √(α × t)

...shows that for concrete, a time of 10,417 seconds corresponds to the "half the temperature increase" point being at a distance of 0.954 × √(2.14×10^-7 × 10,417) = 0.045 m.

(To obtain the value of x, 0.045 m, we have t = 1.1 × x² / α, where α is 2.14×10^-7 m²/s, and t = 52.5 MJ / [0.3 W/m.K × 1.64 m² × (625 - 164 °C) / x meters]. Thus, this simplifies to a quadratic from which distance x can be easily solved.)

Where the column is not touching the concrete dust, it radiates its heat away according to the Stefan-Boltzmann law of radiative heat transfer:

P = A ×e × σ × Te⁴

where P is the rate of radiative heat transfer, A is the exposed area of the emitter (the steel), e is the emissivity, σ is the Stefan-Boltzmann constant of 5.6703×10^-8 W/m².K⁴, and Te is the absolute temperature of the emitter. (We ignore the heat radiated to the steel from the concrete, since this is much lower when the concrete is cold. As the concrete approaches the steel temperature, the net transfer of heat radiated between the steel and the concrete will decrease until it matches the heat conducted away through the concrete.) Eurocode 3 recommends a constant value of 0.625 as the emissivity for steel, where the value for the particular steel at a particular temperature is unknown. Nevertheless, let's assume a low estimate of 0.2.

So we have P = 1.64 m² × 0.2 × 5.6703×10^-8 W/m².K⁴ × 1023⁴ K = 20.37 kW

Thus, even assuming a low emissivity, heat is initially emitted from the steel at a higher rate compared to the conduction case (5.04 kW), and remains so since the heat is conducted away through a larger area of concrete.

Of course, these are only approximations. For example, the thermal gradient through the concrete is non-linear, with the largest gradient at the hot end. But it serves to demonstrate that under the "office fires did it" scenario, a massive steel column would remain cherry-red for hours rather than days or weeks.

Thus, a steel member such as a W14x257, which would be too massive to attain a temperature of 750 °C in a 102-minute office fire, is not massive enough to maintain a temperature of close to 750 °C for six weeks, and the government theory is therefore false. There is no inherent contradiction in that, because six weeks is rather longer than 102 minutes - about 593 times longer. But there is a contradiction in the government's claim that a steel column was heated to cherry-red in a 102-minute office fire and somehow remained cherry-red for six weeks in a debris pile with a mean temperature of 13 °C or lower, or a fire in a debris pile with a maximum possible heat release rate of 1.69 W/m² somehow heated a steel column to cherry-red at the end of October 2001, and the fact that such events are prohibited by the laws of physics.

Cherry-red steel, molten metal, and 'fires' that burned for months are all inconsistent with government claims of an "Arab" conspiracy. But molten iron from a thermitic reaction that was "running down the channel rails" like "you're in a foundry", like "lava", would be the most effective means of transferring heat over large distances within the pile as part of a sequence in which active thermitic material with an ignition temperature of ~430 °C would be ignited and its molten iron product would go on to ignite unreacted thermite elsewhere in its turn, or even unburned combustibles, subject to the constraints of available oxygen.

Government apologists and propagandists for the 9/11 perpetrators tried to create a legend about fires that raged and kept getting hotter because of heat that was trapped in the pile. But if the official story were true, there would never be any mysterious thermal runaway at Ground Zero, in which temperatures kept building up until they exceeded those of office fires. The pile temperatures could not have increased by more than one Fahrenheit degree if the official conspiracy theory were true. The idea that pieces of smoldering combustibles in the ruins of skyscrapers that collapsed from normal "office fires" could significantly heat massive steel beams or even melt aluminum or steel, when the available oxygen could support a power density of no more than one-third of a burning cigarette per square meter, and when the mean temperature in the debris pile should have been about 1 Fahrenheit degree above ambient temperature, is in the realms of nutty conspiracy theories and fringe science concocted by paranoid cranks who spend too much time watching Star Trek.

In conclusion, it is patently obvious that if the government conspiracy theory were true, the WTC underground fires should not have persisted for weeks or months on end, considering the fact that they were drenched with millions of gallons of water and thousands of gallons of fire retardant foam and smothered with tens of thousands of tons of concrete dust. It is patently obvious that molten metal should not have been found if the government account were true. And it is patently obvious that accelerants such as thermitic material could account for molten iron or steel, and for fires that were mysteriously persistent - despite the best efforts of fire-fighters to extinguish them.

What was found at the World Trade Center

4) There were numerous reports of molten steel and molten metal found in the WTC debris pile. Dr. Abolhassan Astaneh-Asl, a professor of structural engineering at Berkeley, saw widespread evidence of extremely high temperatures at the WTC. He saw melting of steel girders, fireproofing that had "melted into a glassy residue", and a wide-flange steel beam from WTC7 with parts of it that had been five-eighths of an inch-thick and had "vaporized" in "searing temperatures". According to Dr. Astaneh-Asl, the beam from WTC7 had burned prior to the building's collapse, and then buckled whilst it was still attached to a column. WTC7 was the building that wasn't hit by any plane that might conceivably have dislodged some of the fireproofing from the steel.

Previously molten metal from the pile was analyzed by independent scientists and found to be abundant in iron. It certainly wasn't an aluminum alloy from the planes.

Source

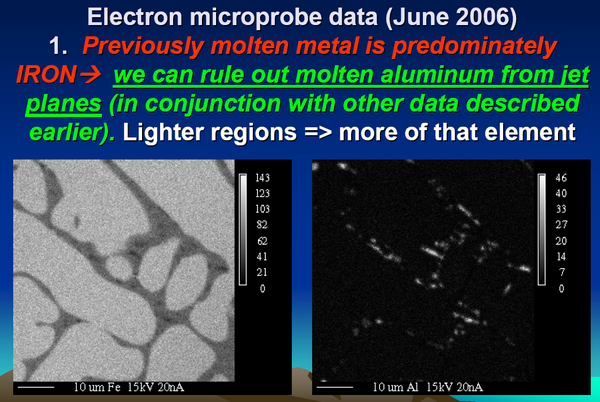
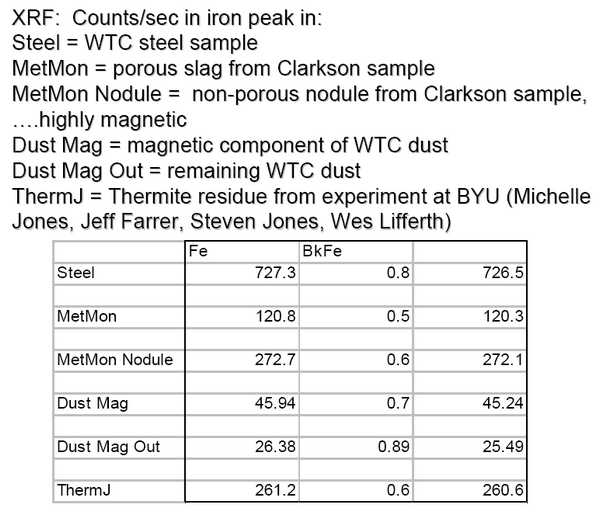
At this point it's worth noting a contradiction between the statements of two NIST engineers: John Gross and S. Shyam Sunder. Dr. Gross, when asked about "pools of molten steel", claimed to be unaware of any such reports. It beggars belief that a lead engineer in a multi-million-dollar study on the WTC collapses could remain ignorant of a whole host of various reports of "rivers of molten steel", a "little river of steel, flowing", "steel [that] flowed in molten streams", and molten "metal" and "steel" that was "running" and "dripping", etc. In a lawyerly way, Gross was playing semantics: the questioner had asked about "pools of molten steel". But if, as is asserted by advocates for the official story, the molten metals found were not steel or iron, then he should have talked briefly about molten "aluminum" or "lead" in order to clarify. Instead, Gross looks like he is being evasive and trying to sweep this very interesting issue under the carpet.

Source: YouTube

(Note how, at the end of the above video, the questioner points out that Gross refused to provide his email address after having invited the questioner to send him thermal imaging data.)

But the intriguing contradiction is in their statements about whether or not steel could have melted. John Gross says [5:09] in the above video, "Steel melts at around 2,600 degrees Fahrenheit. I think it's probably pretty difficult to get that kind of temperature in fire." In contrast, Gross's colleague Dr. S. Shyam Sunder claimed [7:29 in the downloaded audio or about two minutes later if listening on the player] in an interview granted August 21, 2008 (the same day NIST released the draft version of its WTC7 report) and first broadcast three days later for NoLiesRadio.org, "However, when you go to the bottom of the building, after the buildings have collapsed, and you have shielding of the fires due to the rubble pile on top of it, the radiation is contained within the shielded region. And therefore, the temperatures can exceed that which you would see in an office fire, or a hydrocarbon fire when the building was standing and the windows were broken or there was a gash from the airplane, so it's a well ventilated fire. So in the case of a shielded fire it is entirely possible, when the steel was in the rubble pile itself, that part of the steel could have melted."

It's pretty obvious that both Gross and Sunder were well aware that steel had melted at the WTC, and that it should not have melted according to the politically desired conclusion of an "Arab conspiracy". So Gross tries to pretend it didn't happen, and Sunder tries to pretend it could have happened. Apart from closing their eyes, putting their fingers in their ears and listening to the voices in their heads, reality deniers and liars have two main choices: cherry-picking the evidence, or resorting to pseudo-science.

At a Stanford University presentation, Leslie Robertson, who along with the late John Skilling was one of the head structural engineers responsible for designing the World Trade Center, confirmed that he saw "a little river of steel, flowing" in the WTC debris pile. Robertson said, "...when you were down there, you have to remember what was above you; the project was on fire for months. Once we're down at the B1 level and there was one of the fire-fighters said 'I think you'd be interested in this', and they pulled off a big block of concrete, and there was a, like a little river of steel, flowing."

In Volume VI- Issue II October 2001 of The Newsletter of the Structural Engineers Association of Utah, the SEAU President James M. Williams wrote a report on a talk given by Leslie Robertson. Williams' report states: "As of 21 days after the attack, the fires were still burning and molten steel was still running".

Confirmation bias addicts and propagandists for the official legend subsequently attempted to rewrite history by denying Robertson had seen any molten steel at the WTC. They pressured Robertson into saying he couldn't remember talking about molten "steel", despite video evidence of him doing precisely that. Some head-in-the-sand characters not only dismissed the sole surviving WTC lead structural engineer's testimony as a "single anecdotal witness account", but even had the nerve to "do a John Gross" and say that molten steel could not have been seen because it was "impossible". Impossible, that is, if the official legend were true - contrary to whatever S. Shyam Sunder might claim!

5) An orange-yellow flow of tons of molten material was recorded pouring from, as NIST wrote, "the top of a window on the 80th floor of WTC 2, four windows removed from the east edge on the north face". According to NIST, a bright spot appeared just before 9:52 a.m., "followed by the flow of a glowing liquid" that "lasted approximately four seconds before subsiding. Many such liquid flows were observed from near this location in the seven minutes leading up to the collapse of this tower." The building collapsed at 9:59 a.m.

The observation is indicative of an iron-rich melt, consistent with a temperature of around 1,000 °C or more, and totally at odds with the "office fires" scenario. A cooling iron-sulfur melt, e.g. from thermate, would remain at the temperature of its eutectic at around 1,000 °C for some time as it gave up its latent heat of fusion. Office fires, even if capable of sustaining gas temperatures in excess of 1,000 °C for sufficient duration, would not produce molten aluminum at ~1,000 °C, because as soon as the metal became molten it would flow away from the heat source.

NIST and reality deniers claimed the material was molten aluminum, but that has a silvery appearance at temperatures close to its melting point of 660 °C. And NIST admitted that the aluminum alloys from the aircraft would have melted at lower temperatures "between 475 degrees Celsius and 640 degrees Celsius (depending on the particular alloy)".

Most people should be capable of spotting what the molten flow from WTC2 most closely resembles: molten aluminum as seen above, or the molten iron produced from a thermitic reaction as shown below.

Thermite's molten iron product is initially white-hot, turning to yellow and orange as it cools. The WTC office fires would be incapable of melting aluminum at a rate consistent with the observed flow rate from WTC2, and there is a contradiction in the reality deniers' requirements for the aluminum from the plane to miraculously shred itself into "magic bullets" which change course in order to rip the fireproofing from all sides of the steel members, and then to reform into thin plates with a large surface area that settle facing the flames or upper gas layer for maximum absorption of radiant heat.

NIST's engineers are not all color-blind, as evidenced by the fact that NIST published an FAQ that included: "11. Why do some photographs show a yellow stream of molten metal pouring down the side of WTC2 that NIST claims was aluminum from the crashed plane although aluminum burns with a white glow?" In their answer to this question, NIST claimed that "the source of the molten material was aluminum alloys from the aircraft", and deceptively attempted to 'explain' the orange-yellow appearance by postulating molten aluminum mixed with burning organic debris. They said, "Pure liquid aluminum would be expected to appear silvery. However, the molten metal was very likely mixed with large amounts of hot, partially burned, solid organic materials (e.g., furniture, carpets, partitions and computers) which can display an orange glow, much like logs burning in a fireplace. The apparent color also would have been affected by slag formation on the surface."

NIST's claim was refuted when experiments showed that burning debris could not impart an orange glow to all of the molten aluminum.

However, in the interview with NIST's Dr. S. Shyam Sunder referred to in 3) above, Sunder claimed that the molten material was "silverish". This of course is an outright lie, as can be confirmed by anyone who actually watches video footage of the molten material flowing out of WTC2, or looks at the image above. And if NIST had really believed that the material was "silverish" or "silvery", they would hardly have bothered in 2006 to concoct a lame 'explanation' of how the material could still be aluminum when it was not "silverish". An 'explanation' that failed dismally.

Sunder also made a revealing Freudian slip, in this excerpt [at 10:21 into the audio]: "And if you're alluding to the molten metal that was seen on the northeast corner of the 82nd or 81st floor of Tower 2 some minutes before the building collapsed... That was an isolated incident where molten steel - molten material, not molten steel, molten material was seen coming out - it was a silverish, silverish color, molten material. And this is the same floor where the airplane crashed into the building, airplanes are made of aluminum, and of course you also know that the skin of the World Trade Center Towers was made of aluminum."

Rather like Benjamin Netanyahu's admissions that the 9/11 attacks were "very good" for Israel (2001) and that Israel was still "benefiting" from 9/11 (2008), Sunder briefly forgot to spout the official line about molten "material", "metal" or "aluminum". And "isolated incident" is Orwellian Newspeak for something that wasn't supposed to happen but did happen "many" times. NIST said in 2006, "Many such liquid flows were observed from near this location in the seven minutes leading up to the collapse of this tower".

6) A wide-flange beam recovered from WTC7 was found to have undergone an extraordinary corrosion that shocked fire-wise professors. The beam had been reduced from a one-inch to half-inch thickness, with gaping holes, some larger than a silver dollar that left it resembling a Swiss cheese. A liquid eutectic mixture of iron, sulfur and oxygen had severely weakened the beam, and the examiners did not rule out the possibility of the phenomenon having started prior to collapse, thereby accelerating the weakening of the steel structure.

Thermate - thermite with added sulfur to lower the melting point of steel - could explain this. "Office fires" or a smoldering pile of debris certainly cannot, which is why the fire-wise professors were "shocked". The boiling point of sulfur is 445 °C, and the iron-oxygen-sulfur eutectic needs a lot of sulfur, which would have evaporated in any slow sulfidation corrosion attack on the steel. And if gypsum wallboard was the source of the sulfur, it would have been bound up in calcium.

As shown in 3) above, the notion that raging fires in the debris pile could lead to runaway temperatures and cause intergranular melting of steel, even given an iron-oxygen-sulfur eutectic, is inconsistent with scientific and natural law.

FEMA's report described the "severe corrosion and subsequent erosion" of the steel samples as a "very unusual event" with "no clear explanation for the source of the sulfur", and called for further "detailed study". Not surprisingly, given that NIST was in charge of the subsequent investigation, no further study into the phenomenon was conducted.

7) The dust that was generated as the WTC buildings collapsed contained a large quantity of iron in the form of spherical particles, which were up to around 1.5 mm in diameter.

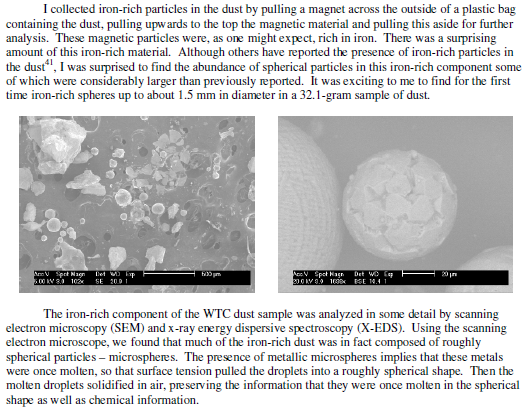
Source: Journal of 9/11 Studies

The RJ Lee Group carried out a damage assessment of the 130 Liberty Street property adjacent to the WTC site, which involved "the most extensive microscopic investigation related to WTC Dust ever performed", including "over 400,000 particles [...] classified using SEM techniques with approximately 80,000 images collected".  They found that the sampled WTC dust was comprised of 5.87% iron spheres, which is nearly 150 times greater than the average 0.04% iron sphere composition in dust collected from typical office buildings.

Source: RJ Lee Group, WTC Dust Signature Report

In the Journal of 9/11 Studies, Prof. Steven E. Jones points out that "the presence of metallic microspheres implies that these metals were once molten, so that surface tension pulled the droplets into a roughly spherical shape. Then the molten droplets solidified in air, preserving the information that they were once molten in the spherical shape as well as chemical information." And the RJ Lee report says that "various metals (most notably iron and lead) were melted during the WTC Event, producing spherical metallic particles. Exposure of phases to high heat results in the formation of spherical particles due to surface tension." 

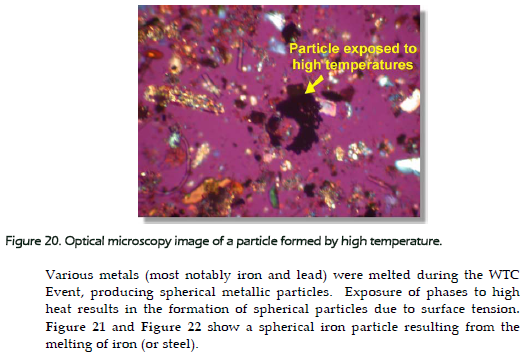
The US Geological Survey (USGS) found iron percentages in the dust to be 4.13, 2.16, 1.41, 1.42, 1.87, 1.87, 1.92, 1.71, 1.49, 2.78, 1.33, 1.72, 1.80, 1.85, and 1.45 from outdoor dust samples, 1.25 and 1.38 from indoor dust samples, and 1.25 and 0.55 from girder coatings; a geometric mean of 1.63%. If we include RJ Lee's findings of 5.87% iron spheres, the mean increases to 1.74%. The USGS mean is more than 40 times greater than that found in "normal" office interior dust.

The above-grade floor slabs in each Tower constituted around 100,000 tons of concrete. Even taking this as a low estimate for the mass of the dust cloud, then 1.74% of 100,000 tons would be 1,740 tons of iron spheres per Tower. If this was the molten iron product of a thermitic reaction, it would need approximately twice that mass of thermite to produce it. Given ~56 tons of fireproofing per floor, if this was switched during the upgrades for a bogus SFRM that was sprayed to twice the original thickness and laced with 50% nano-thermite of greater density than the SFRM it replaced, that would allow more than 56 tons of thermite per "upgraded" floor. So 3,480 tons of thermite requires nearly 62 sabotaged floors, i.e., too many. It would need only five consecutive floors to be taken out - or four if the columns had already been heated by a combination of office fires and a bogus SFRM that rendered them even more vulnerable than wholly exposed steel, for there to be a serious risk of global instability and collapse through removal of bracing that resulted in too many core columns exceeding their critical slenderness value. The targeting of six consecutive floors with, say, 50 tons of thermite per floor, would lead to some 150 tons of molten iron product per Tower. (The thermite was likely divided into incendiary and impulse-managed nano-sized that was tailored for the required balance between percussive effect and attempted minimizing of blast sounds.)

There are sources other than thermite that could account for iron-rich spheres, but these would be of ferric oxide (Fe₂O₃). Thus, most iron spheres would be oxygen rich, and those indicative of thermite would be a minority, possibly around 1 in 10, that contained relatively little oxygen. The spheres evaluated by Steven E. Jones et al included oxygen rich, e.g., their Figure 5 which had an atomic percentage for oxygen of 60 and iron of 39 indicating Fe₂O₃, and iron rich, e.g., their Figure 4 that had 18% oxygen and 65% iron. Figure 4 was the largest spherule from sample 2, which was collected from a fourth floor apartment. Another sample, collected from near the Brooklyn Bridge within twenty minutes of the collapse of WTC1, included spherules as per their Figures 1 to 5, ruling out any possibility of spheres originating from the clean-up operations.

Portland cement contains about 3% (0 to 6%) ferric oxide (Fe₂O₃). A typical composition of starting materials for Portland cement manufacture is lime 68%, silica 22%, alumina 5%, iron oxide 3%, and other oxides 2%. In the cement manufacturing process, the raw materials are crushed and mixed homogeneously, and then fed into a rotary kiln to form the clinker. The kiln heats the mixture to ~1,450 °C although the flame temperature is said to be 2,000 °C, and the materials combine to form new compounds such as ferrites of calcium. If unreacted Fe₂O₃ exceeded its melting point of 1,565 °C and was dispersed into an aerosol of molten droplets within the kiln then it could conceivably solidify into spherules, which would be too improbable to account for a significant percentage. But the bulk of the mix to form the concrete is aggregate. In a typical example (below), the cementitious material comprises some 16.4% of the mix, so even the maximum 6% ferric oxide would amount to less than 1% of the concrete. Any spheres would be a small proportion of that, and they'd be oxygen rich.

Iron and steel slags are used in concrete aggregates, but these tend to be for road construction and railroad ballast. Steel slag is "not recommended for use in rigid confined applications" such as "floor slabs". Manganese, iron and sulfur compounds could make up a few percent of the composition of iron and steel slag. If used in mineral wool, that would comprise a relatively small fraction of the WTC dust.

Fly ash, used as a substitute for up to 50% of the cementitious materials in a concrete mix, is a known source of iron oxide spheres. Early use of fly ash in concrete applications tended to be for structures such as dams rather than buildings, and after the 1970s it was increasingly used in buildings. In 1983 the government issued guideline encouraging its use in federal projects, and in the 1980s the Washington, D.C. area Metro subway system was built using more than 200,000 cubic yards of concrete containing coal fly ash. There is no confirmation either way as to whether or not the concrete for the WTC floor slabs contained fly ash. However, the new One World Trade Center is confirmed as having a "greener" fly-ash concrete, supplied by Eastern Concrete Materials Inc. An October 31, 1968 report of Engineering News Record confirms that "a mixture of high-early strength cement, water and fly ash" was used to grout sockets in the Bathtub, for a system of exterior anchors that was designed to support the slurry wall until the sub-grade reinforced floors were in place to take over the job.

Class F fly ash can have as much as 19% iron oxide. The greatest proportion of iron oxide would be found in a high-volume fly ash (HVFA) concrete featuring Class F fly ash. An example would be coarse aggregate 1,089 kg/m³, fine aggregate 726 kg/m³, type 1 Portland cement 177 kg/m³, Class F fly ash 178 kg/m³. Thus, the fly ash is ~50% of the total cementitious material and 8.2% of the 2,170 kg/m³ concrete mix. If 19% of the fly ash is iron oxide, then 1.56% of the concrete mix is iron oxide. If 1.56% of 100,000 tons of dust is iron oxide, making 1,560 tons, and 150 tons is elemental iron from thermite, then the iron total is 1.71%, which is not far off the mean 1.74% from the USGS and R J Lee reports.

That doesn't count iron oxide from the cement. But given that the concrete for the WTC floor slabs was poured in the late 1960s, which is relatively early in the history of fly ash as a cementitious substitute in concrete in buildings and long before the modern-day predilection for recycling and propaganda about so-called "global warming", a 50% proportion of fly ash would be unlikely. If the cementitious material is 16.4% of the total including aggregate, and three-quarters of the 16.4% is cement with 6% iron oxide, then that iron oxide comprises 0.738% of the total. With fly ash replacing one-quarter of the 16.4% cement, even if it has 19% iron oxide, which is high even for Class F fly ash, that would account for another 0.779%, making the total ~1.5% . Those figures of 6% iron oxide for cement and 19% for Class F fly ash are both at the high end of the ranges, and more typical values would be 3% and 10%. Other sources such as primer paint would account for some iron oxide, but the contributions from these sources would be low compared to the concrete.

The Steven E. Jones team had a sample of WTC concrete that they crushed and looked for iron spheres. None were found. It's possible that most of the concrete contained iron spheres and the Jones sample was a small, unrepresentative sample that happened to have no iron. But that is unlikely. Even if the spheres were in the normal weight but not the lightweight, say, it would be unlikely to account for the observed 1.74% of the dust. Another possible source of Fe₂O₃ is unreacted thermitic material that was prepared using the sol-gel methodology.

So, the big discrepancy between the 0.04% iron sphere composition of "normal" office interior dust and that found in the WTC dust might be explained by the fact that the WTC collapsed and the concrete was crushed, in contrast to the "normal" office buildings. But iron-rich spheres with low oxygen content are indicative of previously molten steel or elemental iron; therefore thermite.

8) An April 2009 paper in the Bentham Open Chemical Physics Journal described red/gray chips found to be ubiquitous in the dense dust that settled over Manhattan following the collapses of the Twin Towers. Apart from an abundance of iron spheres as mentioned above, the dust contained distinctive red-gray chips, which produced iron spheres on heating - evidence that a thermite reaction had occurred.

Source: Nanoenergetic Materials for MEMS: A Review Rossi et al (2007)

A paper by Rossi et al shows how research into nano-thermite was being conducted years prior to 9/11/01. For example, the paper tells of work by Aumann et al on a molybdenum trioxide-based aluminothermic nano-composite as early as 1995. A stoichiometric mixture of this material had an energy density by mass about 12% greater than TNT, and given that the Al/MoO₃ mixture was a little more than twice the mass density of TNT, the Al/MoO₃ reached 16 kJ/cc. The mixture reacted "more than 1,000 times faster than conventional [macroscale] powdered thermite". Interestingly, FOIA requests by the authors of the 2008 paper Extremely high temperatures during the WTC destruction revealed that the USGS study had found a molybdenum-rich spherule (indicative of a previously molten state) in the WTC dust. The spherule also contained some aluminum, oxygen and calcium, with the XEDS plot showing the aluminum peak 5 times lower than the dominant molybdenum peak. Molybdenum has a melting point of 2,623 °C (4,753 °F), although that could be lowered by the addition of other elements.

NIST shies away from peer-review of its own work. It refuses to provide details of the input parameters for its computer models of the WTC7 fires, on the grounds that such release would "jeopardize public safety". It's not "public" safety that they are concerned about; it's the safety of Sunder, Gross, Grosshandler, Gann, Bukowski et al!

9) The "loaded gun" described in 8) - active accelerant - provides an explanation for the "smoking gun" observations 4) to 6) and possibly 7), which cannot be accounted for in the official conspiracy theory 1) without abandoning scientific laws described in 2) and 3). Apart from on 9/11, no other steel-framed high-rises in the U.S. have ever collapsed from fire, aircraft impact, "thermal expansion" of fireproofed steel members, or a combination thereof. Office fires fueled by carpets, workstations and paper burning in air are incapable of melting iron and steel, and do not leave tons of highly energetic nano-engineered active thermitic material in dust clouds. Technicians in the controlled demolition industry are well aware that "Fire cannot bring down steel-framed high rises -- period", that there is no need for "miles of det cord" in a high budget project since wireless detonation is available, and any cutter charges would have self-consuming casings that left only molten iron. However, deceptive demolitions designed to resemble building collapses effected by aircraft impact and ensuing fires would be expected to leave the reactants and the products of a thermite reaction - with clear physical evidence of very high temperatures. And sure enough, this is precisely what happened. The scientific debate on controlled demolitions at the WTC is over, with fire-induced collapses not even a vague possibility.

Did "the Arabs" do 9/11?

10) Israel's fingerprints are all over the WTC attacks. And right from the start, the plan was for the WTC to be "used by U. S. and foreign governmental agencies".

Source: Engineering News Record, April 13, 1967

The foreign governmental agency of relevance here is that of a certain country the size of New Jersey, created in 1948 on the back of Zionist terrorism such as the King David Hotel bombing (1946) in which Jews dressed as Arabs, prior to classic examples of false-flag terror operations by the Israeli state, e.g. the Lavon Affair (1954). It's the state whose intelligence agency was caught in 2002 attempting to create a phony "Palestinian al Qaeda" cell in the Gaza Strip, the motive being to provide a pretext for Israeli attacks on Palestinian civilians. In 1991, Israeli security officials inspected the garage of Zim American Israeli Shipping in the WTC and concluded that the WTC garage would be vulnerable to a car bomb. Two years later, the WTC garage was hit by a car bomb, and Israeli intelligence sources admitted that Ahmad Ajaj, involved in the early stages of the plot, was a Mossad mole. The 1993 bombing provided the 9/11 planners with the pretext they needed for "fireproofing upgrades" at the WTC. After 9/11/2001, it was found that there was a remarkable correlation between the impact floors of each Tower that supposedly failed due to "fires", and those floors that had received fireproofing "upgrades", particularly in the case of the North Tower (WTC1). (For those who still haven't figured it out, the Zionist perpetrators, not "Arab hijackers", were steering the planes.) And the Zim American Israeli Shipping Company moved out of the WTC on September 4, 2001, transferring their HQ to Norfolk, Virginia. That was five weeks after Larry Silverstein - a close friend of Benjamin Netanyahu, Ariel Sharon and Ehud Barak - and Frank Lowy - a friend and business partner of Ehud Olmert - had acquired a 99-year lease on the entire WTC complex. In February 2009 it was revealed that Ali al-Jarrah, whose cousin Ziad al-Jarrah was named as one of the alleged nineteen 9/11 hijackers, received more than $300,000 for his "work" as a paid spy for Israel going back to 1983 whilst posing as a supporter of the Palestinian cause.

Israel is by no means the only state that employs false-flag terror for political purposes. But from a plenitude of evidence far too copious to delve into in this report, it is established beyond the slightest shadow of doubt that Israel's agents had foreknowledge of the 9/11 attacks. However, this was not simply a case of Mossad agents shadowing "Muslim hijackers" and letting the plot go ahead. All the other corroborating evidence of Israel's central role as the principal perpetrator of 9/11, together with the proof of controlled demolitions as presented in 1) to 8), shows that a power-crazed Zionist ruling elite did not merely "LIHOP" - let the 9/11 attacks happen on purpose. They made it happen on purpose and made damn sure that the Twin Towers collapsed killing thousands of people, so that Israel's so-called "allies" would be sufficiently outraged to wage war on Israel's foes. See The "Dancing Israelis" FBI Report - Debunked and Daily Mail 'proves' fire brought down WTC7 for full analysis and links to sources.

Evidence that is supposed to support the official conspiracy theory

Given the overwhelming evidence against the official 9/11 conspiracy theory that claims that the Arabs did it, the next step is to weigh all this evidence against that which supports the official theory. So let's see...

11) Planes hit buildings, then buildings came down. This is one of the reality deniers' favorite 'proofs'. They like to overlook the fact that only two buildings were hit by a plane, yet three buildings completely collapsed like a house of cards.

Correlation is not proof of causation. The 9/11 conspirators staged plane crashes followed by controlled demolitions. The latter were to ensure that the buildings came down in order to effect sufficient outrage and desire for revenge; the former were to make people fall for the logical error of post hoc ergo propter hoc (after this therefore because of this) that confuses correlation with causation, and imagine that the planes were responsible. Selling the concept of "Muslim suicide pilots and hijackers" who "got lucky" was easier than selling a yarn about Muslim terrorists who'd managed to plant explosives that brought down three skyscrapers. Had the Flight 93 take off not been delayed for 41 minutes due to congestion, it probably would have hit its target (WTC7) - rather than getting shot down and leaving the conspirators with a building that was wired for demolition, but with no plane crash to provide the pretext. Thus, NIST had to spend years concocting a 'theory' about "thermal expansion" (the NIST employee who came up with the notion of "thermal expansion" as an 'explanation' did at least get the idea from reading a report on the Cardington fire tests, but that was the easy part). And even then they didn't dare publish it until after receiving confirmation of the death of eyewitness Barry Jennings in 2008.

12) A "hijacker's passport", allegedly of Satam al-Suqami (although some reports claimed it was Atta's), is said to have been "discovered" before the Twin Towers collapsed. The mysterious civilian who "discovered" it handed it to a detective and ran off, never to be seen again. The passport is supposed to have inexplicably survived the fireball and floated 1,200 feet down to the ground almost completely unscathed, despite being "soaked in jet fuel".

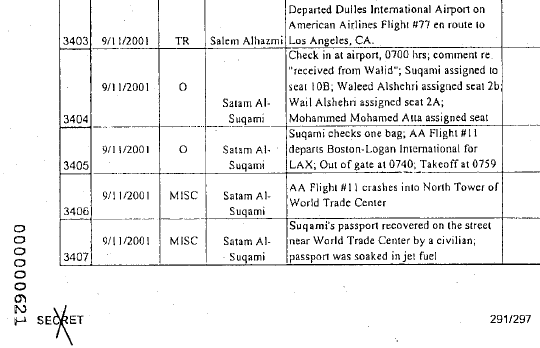
Source: FBI redacted document, page 291

Bizarrely, the jet fuel that "soaked" the passport didn't ignite, but the rest of the 10,000 gallons did ignite, forming a huge fireball and igniting office combustibles on several floors of the WTC. Moreover, of the various locations claimed for this "discovery" - in the vicinity of Vesey Street, a few blocks from the crash site, and several blocks from the crash site - not one of them is consistent with the laws of aerodynamics. Suqami was supposed to be on Flight 11 which approached from the north, but Vesey Street is to the north of WTC1. The passport should have either hit the northwest facade of WTC2 and wound up within the WTC site, or, had it cleared the side of WTC2, might have landed in Liberty Street to the south. Drag forces would have rapidly decelerated it before it could clear buildings to the south of Liberty Street in order to end up "blocks" away, and the overpressure from the hydrocarbon deflagration was insufficient to reverse its forward velocity and propel it northwards back to Vesey Street.

Then there is the oddity of the passport supposedly being "soaked" in jet fuel, yet the jet fuel was atomized into a fine mist - which is how it was able to ignite whilst cooler than the minimum flash point of 38 °C for kerosene-grade Jet A fuel. Thus, the passport should have been slightly damp rather than soaked, even if unignited jet fuel had caught up with it. The passport is supposed to have a sufficiently high initial velocity whilst dodging the core columns, partitions, perimeter columns, filing cabinets, etc., and exiting the North Tower, coupled with a sufficiently high mass compared to the product of its mean frontal cross-sectional area (as it spins) and its drag coefficient, in order to end up "blocks" away. Yet any unignited, atomized jet fuel droplets that improbably outran the fireball, like the passport was supposed to have, would have a comparatively low density and thereby would undergo greater deceleration from air resistance, so that the jet fuel would never catch up with the speeding passport to dampen it slightly, let alone "soak" it.

The "soaked in jet fuel" story sounds like it was concocted by the same inept scriptwriters who fabricated the passport story in the first place, making such a hash of the job that they didn't even bother to check which plane the framed Suqami was supposed to have been on. And the passport was said to have been "clearly doctored", i.e., "manipulated in a fraudulent manner in ways that have been associated with al Qaeda", or at least, with whoever wants the public to buy into the "al Qaeda" conspiracy theory.

It transpires that the New York Police Commissioner who announced the 'discovery' was subsequently convicted and sent to jail for lying, conspiracy and fraud. He made a four-day trip to Israel two weeks prior to 9/11 (August 26 - 29, 2001), where he met an Israeli billionaire. It was later found that this bent police chief had received a $250,000 "loan" from the very same Israeli billionaire after claiming the passport had been discovered, and had also benefited to the tune of $236,269 in rent paid by another Jewish businessman over the two years following 9/11 from December 2001 to December 2003.

Interestingly, Bernard Kerik's boss at the time, Rudy Giuliani, who banned photography at Ground Zero, was in London on the morning of the 7/7 bombings of 2005, and visited Yorkshire in northern England the day before the bombings. In an amazing 'coincidence', a till receipt obtained from a garden center at Tulip Retail Park in Leeds, Yorkshire was 'found' at the site of one of the deadly Tube train blasts after reputedly surviving the explosion, and was cited as 'proof' that the Muslims from Yorkshire were responsible for the attacks. In another 'coincidence', Benjamin Netanyahu was also located in London on the morning of the 7/7 bombings, of which Netanyahu and the Mossad had foreknowledge. Netanyahu, who wrote in a 1995 book about "militant Islam" bringing down the "World Trade Center", and who declared on separate occasions that 9/11 was "very good" and beneficial for Israel, and who served in the clandestine Israeli military elite special forces unit Sayeret Matkal, is one of the Israeli prime ministers who were close friends of Larry Silverstein. The latter not only insured the WTC for $billions against terrorism when taking over the lease six weeks prior to 9/11, but also 'coincidently' failed to turn up for work on 9/11, along with his two children.

Daniel Mark Lewin, an Israeli-American who was on Flight 11, was ex-Sayeret Matkal and an alumnus of MIT, as was Netanyahu. Many Sayeret Matkal members were originally Aman mista'arvim - experts at disguising themselves as Arabs. The official story claims that Suqami was seated behind Lewin and killed him. An "Arab" hijacker armed with a "box cutter" manages to kill an ex-military elite special forces guy, and other "hijackers" such as the diminutive Hani Hanjour manage to convince at least three pilots such as the tough, highly trained, weightlifting former Navy fighter pilot Charles Burlingame to hand over control of their planes??? In yet another remarkable 'coincidence', another alleged "hijacker" on Flight 11 was Abdulaziz Alomari, and Lewin was a native of Denver, which is where one of the "wrong" Alomaris had his passport stolen. The Mossad, of course, has a well-deserved reputation for stealing and faking passports and identities, as in New Zealand and Dubai, and when they posed as American CIA agents in an attempt to recruit Jundallah militants in another false-flag.

In the arena of Zionist false-flag terror operations, it's a small world, but a vast, complex web of lies and deception.

A good exposition of the frame-up and the evolving list of hijackers' names can be found in Paul Zarembka's The Hidden History of 9-11.

Source: Paul Zarembka The Hidden History of 9-11

13) It is often claimed that Osama bin Laden admitted responsibility for 9/11. A well-built, dark-skinned, right-handed man, dubbed informally as "Fatty bin Laden", whose only resemblance to the pale, olive-skinned, 6' 4" to 6' 6", approximately 160 lbs, left-handed Osama bin Laden is that they both sported a beard and wore a turban, appeared in a video that was conveniently 'found' in Jalalabad, a city with a population estimated at roughly 250,000 as of 1999. The chubby, right-handed individual admitted responsibility for 9/11 after Osama bin Laden, who died December 2001, had denied responsibility on at least two occasions: September 16 and 28 of 2001. Various tapes of "Osama" were faked in the ensuing years, often merely consisting of audio, and sometimes accompanied by a still image. The tapes were broadcast at times that would help the Bush admin, such as days prior to the 2004 presidential election. The Washington Post reported in May 2010 that former CIA officers admitted the agency created tapes in which their "darker-skinned employees" acted the roles of Osama and his cronies.

The Al Jazeera channel, which broadcast audios and videos purportedly of Osama bin Laden, was started in 1996 with a $150 million grant from Sheikh Hamad bin Khalifah Al-Thani, the Emir of Qatar. From October 2 through to October 5, 2001, the Emir visited the U.S. where he met with George W. Bush, Dick Cheney (an "old friend"), Colin Powell, Rudy Giuliani, Paul Wolfowitz and Richard Myers. On October 20, Dick Cheney again met his friend the Emir of Qatar to discuss the "Osama" interviews.

On May 1-2, 2011, U.S. forces murdered several members of the Khan family of House No 3, Street No 8-A, Garga Road, Thanda Chowa, Hashmi Colony, Abbottabad, Pakistan in cold blood, claiming that Akbar, the uncle of Arshad and Tariq, was Osama bin Laden. Akbar held the TV remote with his right hand in contrast to the real Osama who was left-handed. And Akbar had a gray beard and wore a beanie hat, in contrast to the black-bearded turban-wearing "Osama" who appears in videos allegedly made in 2007. The new legend held that Akbar / "Osama" dyed his beard black and wore a turban for his "broadcasts", although even this ad hoc 'explanation' failed to account for "Osama's" conversion to a right-handed man.

Source: The Telegraph

Source: Daily Mail

Arshad was claimed to be Osama's "courier". The government story kept changing. For example, first it was claimed that "Osama" was armed, and then it was admitted that he was unarmed, but the killing was said to be justified on the grounds that "Osama" had "resisted during the raid". After the killings, two buffalos, a cow and around 150 chickens were removed from the 13-room compound. Initially the government claimed to have had live feed of the attack and provided a photograph of Obama admin members in suitably anxious-looking poses to 'prove' it, but later admitted that the live feed was blacked out for 25 minutes during the raid. It turned out that Barack Obama had been playing golf until 20 minutes before "the operation began in earnest".

Akbar's body was "buried" (dumped) in the sea in order to destroy the evidence, and an unsupported claim about "DNA testing" served to 'prove' the body was that of Osama. After a two-year-old image of a dead "Osama" was broadcast on Pakistani TV channels and websites of Western mainstream news outlets, and then exposed as a composite of Osama from 1998 together with the corpse of an Iraqi who'd been killed after being arrested in Baghdad in February 2006, no further images were officially released. Rather than risk being caught again with yet another fake, it was asserted that publication of such a "graphic" image would be "too provocative" and would threaten "national security". (As if killing the unarmed, alleged leader of an international terrorist organization and dumping his body in the sea wasn't already provocative enough???) A chopper 'accident' conveniently provided a pretext for avoiding bringing any "Osama wives" back to the U.S. for interrogation, on grounds that there was "no room". A New York Times article dated May 1, 2011, (just hours before the butchering of the Khan family) on how U.S. aid to Pakistan was in excess of $1 billion annually, provided a thinly veiled threat as to what would happen should the Pakistani authorities put up more than a token resistance to the U.S. version of events.

In August 2011, 30 American Special Forces troops and eight Afghan soldiers were killed when a helicopter was shot down over Afghanistan. The thirty Americans included 22 members of the SEAL Team Six that killed the Abbottabad resident alleged to be Osama bin Laden. The government claimed that the 22 SEALs who died in the helicopter crash of August 2011 "were not among the 23 who killed Bin Laden" in May 2011. The names of those killed were published, but the names of those who carried out the Abbottabad killings were not released, and so the government's claim cannot be verified. However, it was confirmed that "the 23 SEALs who killed Bin Laden at his compound in Pakistan in May had recently returned to Afghanistan from their base in North Carolina". No body, no "Osama" wife, and possibly no remaining executioners. The government would likely describe the Team Six deaths as a tragic "coincidence", rather like they described officials at the National Reconnaissance Office, Chantilly, VA, starting to run an exercise involving a plane crashing into a tower at 9:00 a.m. on 9/11/01 as a "bizarre coincidence".

An analogy

(Some details have not been changed, since that would render them too obscure.)

Three famous New Yorkers are found lying dead in the street. Two of them have superficial gunshot wounds to the arms, and the other has a minor bruise. Although it is freezing outside, all three bodies are still warm. The government alleges that the gunshot wounds and bruise were the cause of death and the killers were from a highly feared, vicious gang that calls itself "The Database", also occasionally known as "The Toilet". Strangely, it is the first time anyone has ever heard of "The Database", although Lynn Badun, the alleged gang leader, has already been blamed by the government for other violent crimes. Database members are said to have been at the crime scene, but certainly no later than five months before the discovery of the victims, which is inconsistent with the temperature of the bodies. One of the autopsies, which is not to be published for another seven years, and only then on a date that happens to be two days after the death of a key witness whose testimony blows apart the government account, will conclude that the victim died from "thermal expansion" of his backbone - after early speculation about methane fires and spontaneous combustion. Upon impact, the bullets are mysteriously shredded into tiny pieces that completely shred all of the victims' clothes, allowing them to become sunburnt in the freezing, overcast conditions. The bullets mysteriously rearrange themselves into thin plates that absorb so much energy from the cloud-obscured sun that pools of molten metal are seen running, flowing and dripping at the crime scene.

One year before the hit job, members of a rival gang known as Project for a New Autocracy of Cruelty (PNAC) authored a paper about the 'benefits' of a "new Pearl Harbor". Six years before the hit job, PNAC's mentor wrote that militant opponents of PNAC would murder the famous New Yorkers. PNAC's mentor was prime minister of a racist, lawless pariah state with a history of staging terrorist attacks and blaming them on another group for political gain - aka false-flag terror. (The regime would go on to commit piracy and murder of humanitarian volunteers in international waters, use of a bulldozer as a lethal weapon to murder a peace activist, attempting to steal a wheelchair-bound cerebral palsy victim's passport, war crimes involving white phosphorus and cluster bombs against civilians, attacks on Red Cross ambulances and a UN observer post, murder of peach-picking farm workers on a pretext about a building being used to store weapons, murder of at least 28 civilians including 16 children by blowing up a three-story building on a pretext about stopping rocket attacks, shelling a picnicking family on a beach as a preliminary tactic when aiming to start a war and then trying to shift the blame, attempting to blow up the Congress of a country whose population had shown very little support for a war that had been launched three days previously, having motorcyclists with magnetic bombs or hit squads dressed as tennis players murder its opponents, etc.) The PNAC mentor temporarily retired from politics a couple of years prior to the hit job, giving him time to orchestrate such an operation; he would go on to serve a second term as prime minister. And as finance minister, he would be on scene in London on the morning of a false-flag terror operation, and would be revealed as having foreknowledge of the London attacks.

The cousin of an intelligence asset who, it later transpired, was paid more than $300,000 over 25 years for his 'work' spying for the pariah state, was at the crime scene at the time of the killings. So was another individual who served in the same elite Army special forces unit and is an alumnus of the same university as the PNAC gang's mentor. Forensic pathology subsequently determines that several lethal military-grade poisons were present in the victims' bodies; the PNAC gang had access to these poisons but the "suspects" did not. The government claims that The Database's motive was a hatred of freedoms and Western culture, but the "suspects" were seen drinking, gambling, paying for escorts and lap dancers, watching pornographic videos and doing cocaine shortly before the killings. Interestingly, PNAC gang members are heavily into drinking, gambling, womanizing, etc., suggesting that PNAC members were mixing business with pleasure whilst posing as The Database members. In contrast to PNAC members, the "suspects" have very poor hand-eye co-ordination and are hopeless with guns; in fact, one of them was even refused permission to play with a water pistol unaccompanied. It is claimed that all "suspects" shot themselves dead, but none of the bodies are ever found, and within two or three weeks half of them turn up alive and well. Without elaborating, the government cites "mistaken identity" as the 'explanation' for this contradiction. The 'evidence' cited for the "Database did it" allegation includes a claim by a former local police chief that a passport from one of the "suspects" was "discovered", almost completely unscathed, after a bullet sent it flying through the air. The passport's alleged trajectory turns out to be in contravention of the laws of aerodynamics. The ex-police chief is later convicted of lying, conspiracy and fraud, after it is found that he received a $250,000 "loan" from a billionaire businessman from the aforementioned racist, lawless, false-flag terror-staging pariah state, and had $236,269 in rent paid by another businessman who received an award from the pariah state a month after the hit, after the ex-police chief claimed that the passport had been "discovered". Two weeks prior to the killings, the ex-police chief made a taxpayer-funded official trip to the pariah state, whereupon he met with the same billionaire who "loaned" him the $250,000. Officially, the trip was supposed to be about fighting terrorism and Ecstasy trafficking, yet the ex-police chief was not accompanied by any of his advisors.

Agents of the pariah state are found to have been at the crime scene, videotaping the killings, high-fiving, smiling, joking and horsing around with cries of joy and mockery, and filming themselves flicking a lighter with the bodies in the background like they were at a rock concert. After being arrested on suspicion of involvement in the murders, each agent provides an entirely different alibi as to how they came to be at the crime scene. None of the alibis is physically possible, given the time it would take for these agents to learn of the killings, gather up their cameras, leave their workplace, borrow their employer's van, and drive through rush-hour traffic. The agents later admit that their job was to "document the event".

Six weeks prior to the murders, multi-billion-dollar life insurance policies are written, insuring the victims. Two close friends of four of the pariah state's prime ministers are the named beneficiaries, and are also responsible for the security of the victims. Another series of murders and criminal damage in Arlington, VA, committed on the same day as the New York slayings, targets bookkeepers, accounting documents and computer systems at a multi-trillion-dollar business that recently needed to make almost $7 trillion of accounting corrections to balance its books, with $2.3 trillion of those adjustments lacking receipts. A member of PNAC, who co-authored the group's paper about a "new Pearl Harbor", is chief accountant at this multi-trillion-dollar business, having landed the job a few months in advance of the hit when the trillions of accounting corrections had already been public knowledge for months, and also has access to technology that can remotely deliver lethal poisons using a Command Transmitter System....

Conclusion

A few years ago, those still living in denial were claiming there were no structural engineers, architects, physicists, chemical engineers, mechanical engineers, metallurgical engineers, PhD scientists, fire protection engineers, fire-fighters, electrical design engineers, explosives technicians, etc., who doubted the official conspiracy theory of how "Osama bin Laden" supposedly pulled off 9/11. Now all of those professionals may be seen on AE911Truth.org's YouTube channel explaining why they know the official story is a pack of lies. Various experts at an international hearing comprehensively debunked the government's conspiracy theory. Plenty of highly credible people have already spoken out against the government version of events. And psychologists explain why some individuals exhibit an irrational resistance to alternative accounts.

All impartial researchers who honestly study the facts will inexorably arrive at the same conclusion. It's a slam dunk. The Twin Towers and WTC7 were destroyed in controlled demolitions. 9/11 was a Zionist false-flag operation.

Index

Air flow through debris pile, more than 1,000 times lower than that required to sustain smoldering combustion

Air required to heat pile to 600 °C, volume of air is 636 times the volume of pile to be heated

Cardington fire tests, no collapse ensued

Cherry-red steel columns, would cool in hours, not weeks

Combustibles, pulverized, would burn up within hours, minutes or seconds

Cortlandt Street/WTC IRC station and subway, heavy damage from collapses but no evidence of smoke or flames

Darcy's law, predicting total discharge of a fluid through a medium

Disinformation: "beam weapons", "nukes", "no planes", "TV fakery", etc

Energetic nanocomposite, Al-Fe₂O₃-based, using nanowires, ignited at 410 °C and produced flame temperature of ~4,000 °C

Experts - structural engineers, architects, physicists, mechanical engineers, etc., - who tear apart the claims of fire-induced collapses

Gross, John, (NIST), denies existence of molten steel

Ground Zero pile, air porosity was 0.1

Ground Zero pile "fires", conclusion

Ground Zero pile "fires", five months of continuous smoldering could heat the steel and the concrete by 1 °F

Ground Zero pile, implausibility of fires burning for five months even if oxygen was available

Ground Zero pile, mean temperature should have been 13 °C (or 3 °C in winter), even if oxygen was available

Ground Zero pile, molten metal analyzed, found to be abundant in iron, very little aluminum

Iron spheres, low oxygen, found in dust, indicative of thermite

Israel, fingerprints all over the WTC attacks

Jet fuel, all burnt up within minutes

Melting points, iron, iron(III) oxide, and structural steel

Molten steel or iron, found at Ground Zero

Molybdenum trioxide-based aluminothermic nano-composite, was being researched in 1995

NIST, refused to provide input parameters for its computer models, claimed release of the information would "jeopardize public safety"

NIST, sponsored tests on WTC-style floor assemblies, no collapse ensued

Nuclear demolitions, disinformation

Passport, "hijacker's", allegedly "discovered" near the WTC site

Permeability of WTC debris pile, is in the same range as sand

Pyrocool FEF fire retardant foam, used at Ground Zero, extinguished oil tanker fire in twelve-and-a-half minutes

Robertson, Leslie, saw "a little river of steel, flowing" in Ground Zero pile

Smoldering, self-sustained, minimum heat flux

Smoldering block in debris pile, comparison with human buried alive

Steel beam was "dripping from the molten steel", five months after 9/11

Steel beams would "burst into flames"

Sulfidation of WTC7 steel beam, no calcium (indicating source was not gypsum), sulfur would have evaporated in any slow corrosion attack

Sunder, Shyam S., (NIST), claims steel "could have melted" in the pile

Sunder, Shyam S., (NIST), claims molten metal pouring from WTC2 was "silverish"

Thermal diffusivity of pile, insufficient to sustain ignition of adjacent fuel for months on end

U. S. Air Force, researched iodine-based thermite, detonation shock wave of ~2 km/s, heat release of 6.22 kJ/g

Underground coal fires, comparison with Ground Zero pile

Water, four million gallons percolated through Ground Zero debris in first ten days after collapses

White smoke in debris pile, inconsistent with smoldering office combustibles

WTC collapses, burning fuel likely to be extinguished by more than ten seconds of crushing, shredding, pulverization, scattering and smothering

WTC fire load, 4 psf combustibles or 8 psf of wood equivalent fuel

WTC fire load released over 3 months, power density would not even meet the minimum definition of sunshine

WTC, housed "foreign governmental agencies"

WTC office fires, gas temperatures

WTC2, flowing molten metal was iron, not aluminum

Further information on whodunit:

Why 9/11 researchers know the official story is false

The "Dancing Israelis" FBI Report - Debunked

Stranger Than Fiction

The Synagogue of Satan

Waters Flowing Eastward

Daily Mail 'proves' fire brought down WTC7

It Wasn't Muslims